Question

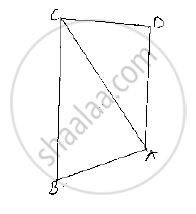
In Fig. 10.131, prove that: (i) CD + DA + AB + BC > 2AC (ii) CD + DA + AB > BC  

 

Answer 1

Given to prove 

(1) CD+DA+AB+BC>2AC 

(2) CD+DA+AB>BC 

From the given figure,
We know that, in a triangle sum of any two sides is greater than the third side 

(1) So,
In ΔABC,we have 

AB+BC>AC                    ...............(1) 

In , ΔADC we have 

CD+DA>AC                 ...............(2) 

Adding (1) and (2) we get 

AB+BC+CD+DA>AC+AC 

⇒ CD+DA+AB+BC+>2AC 

(2) Now, in , ΔABC we have  

AB+AC>BC                      .............(3) 

and in , ΔADC  we have 

CD+DA>AC 

Add AB on both sides 

⇒ CD+DA+AB>AC+AB ............(4) 

From equation (3) and (4), we get 

CD+DA+AB>AC+AB>BC 

⇒CD+DA+AB>BC 

∴ Hence proved