Advertisements
Advertisements
Question
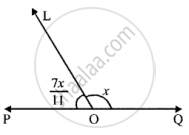
In the case given below, find the value of x so that POQ is straight line

Solution
5.5x + 15° = 180°
⇒ 5.5x = 180° - 15°
⇒ 5.5x = 165°
⇒ `"18x"/11 = 180°`
⇒ x = `165/5.5 = (165 xx 10)/55` = 30°
∴ x = 30°
APPEARS IN
RELATED QUESTIONS
In your note-book copy the following angle using ruler and a pair compass only.

Construct the 90° angle, using ruler and a pair of compass only.
Construct the 45° angle, using ruler and a pair of compass only.
Draw a line segment PQ = 8cm. Construct the perpendicular bisector of the line segment PQ. Let the perpendicular bisector drawn meet PQ at point R. Measure the lengths of PR and QR. Is PR = QR?
In the following figure, AB is parallel to CD; find the values of angles x, y and z:

In the following figures, PQ is parallel to RS. Find the angles a, b and c:
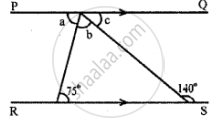
In the following figures, PQ is parallel to RS. Find the angles a, b and c:

Two straight lines are cut by a transversal so that the co-interior angles are supplementary. Are the straight lines parallel?
In the case given below, find the value of x so that POQ is straight line
Without using set squares, construct angle ABC = 60° in which AB = BC = 5 cm. Join A and C and measure the length of AC.
