Advertisements
Advertisements
Question
In the diagram below, PQ is a ray of light incident on a rectangular glass block.
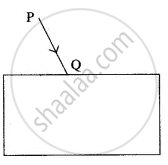
How are the angles ‘i’ and ‘e’ related to each other?
Solution
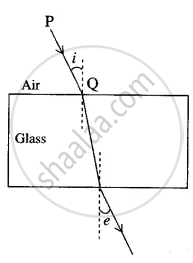
When the incident ray is undergoing minimum deviation the angle of incidence is equal to the angle of emergence, i.e., ∠i – ∠e
APPEARS IN
RELATED QUESTIONS
A student traces the path of a ray of light passing through a rectangular glass slab and marks the angle of incidence i, angle of refraction r and angle of emergence e, as shown.
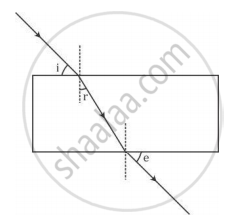
The correctly marked angle(s) is/are
(A) ∠ i only
(B) ∠ e only
(C) ∠ r only
(D) ∠ i and ∠ e
How is the angle of emergence related to the angle of incidence when prism is in the position of minimum deviation? Illustrate your answer with help of a labelled diagram using an equilateral prism?
The refractive index of glass is 1.5. From a point P inside a glass block, draw rays PA, PB and PC incident on the glass air surface at an angle of incidence 30°, 42° and 60° respectively.
- In the diagram show the approximate direction of these rays as they emerge out of the block.
- What is the angle of refraction for the ray PB?
`("Take" sin 42° =2/3)`
(i) What is the relation between the refractive index of water with respect to air `("_aμ_\text(w))` and the refractive index of air with respect to water `("_\text(w)μ_ a)` .
(ii) If the refractive index of water with respect to air `("_aμ_\text(w))` is`5/3`. Calculate the refractive index of air with respect to water `("_\text(w)μ_ a)` .
Define the term refractive index of a medium. What do you understand by the statement 'the refractive index of glass is 1.5 for white light'?
Why does a fisherman aim at the tail of fish during spearfishing?
Draw ray of light bending towards the normal while passing from glass to water. Label your diagrams.
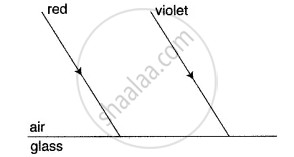
Two parallel rays of Red and Violet travelling through the air, meet the air-glass boundary as shown in the above figure. Will their paths inside the glass be parallel? Give a reason for your answer.
The speed of light in air is 3 × 108 ms-1. Calculate the speed of light in water. The refractive index of water is 4/3.
