Advertisements
Advertisements
Question
In the following figure, BA is parallel to CD. Find the angles a, b and c:
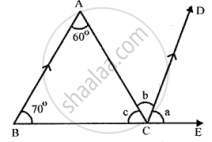
Solution
In the given figure,
ABC is a triangle and CD || BA, BC is produced to E.

∠A = 60°, ∠B = 70°
∵ AB || DC and BE is its transversal
∴ ∠DCE = ∠ABC (Corresponding angles)
⇒ a = 70°
∴ a = 70°
Similarly, AB || DC and AC is its transversal
∴ ∠ACD = ∠BAC (Alternate angles)
⇒ b = 60°
∴ b = 60°
But a + b + c = 180° (Straight line angle)
⇒ 70° + 60° + c = 180°
⇒ 130° + c = 180°
⇒ c = 180° - 130° = 50°
Hence a = 70°, b = 60° and c = 50°
APPEARS IN
RELATED QUESTIONS
The adjoining figure shows two straight lines AB and CD intersecting at point P. If ∠BPC = 4x – 5° and ∠APD = 3x + 15°; find:

(i) the value of x.
(ii) ∠APD
(iii) ∠BPD
(iv) ∠BPC
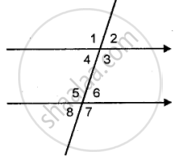
If ∠1 = 120°, find the measures of : ∠2, ∠3, ∠4, ∠5, ∠6, ∠7 and ∠8. Give reasons.
Construct the 90° angle, using ruler and a pair of compass only.
Construct the 45° angle, using ruler and a pair of compass only.
Construct the 30° angle, using ruler and a pair of compass only.
Draw a line segment PQ = 8cm. Construct the perpendicular bisector of the line segment PQ. Let the perpendicular bisector drawn meet PQ at point R. Measure the lengths of PR and QR. Is PR = QR?
Draw a line segment AB = 6.5 cm. Locate a point P that is 5 cm from A and 4.6 cm from B. Through the point P, draw a perpendicular on to the line segment AB.
Two straight lines are cut by a transversal. Are the corresponding angles always
Two straight lines are cut by a transversal so that the co-interior angles are equal. What must be the measure of each interior angle to make the straight lines parallel to each other?
Draw a line AB = 9 cm. Mark a point P in AB such that AP=5 cm. Through P draw (using set-square) perpendicular PQ = 3 cm. Measure BQ.
