Advertisements
Advertisements
Question
In the given figure, l || m || n. ∠QPS = 35° and ∠QRT = 55°. Find ∠PQR.

Solution
We have,
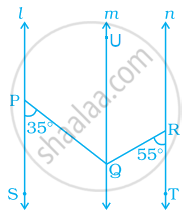
l || m and PQ is a transversal
∴ ∠SPQ = ∠PQU .....[Alternate interior angles]
⇒ ∠PQU = 35° ......(i)
Also, m || n and QR is a transversal.
∴ ∠QRT = ∠RQU ......[Alternate interior angles]
⇒ ∠RQU = 35° ......(ii)
Now, ∠PQR = ∠PQU + ∠UQR
= 35° + 55° ......[Using (i) and (ii)]
= 90°
APPEARS IN
RELATED QUESTIONS
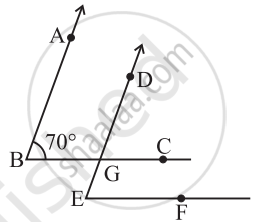
In the given figure, the arms of two angles are parallel.
If ∠ABC = 70°, then find
- ∠DGC
- ∠DEF
Find the measure of angle y in the following figure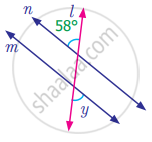
Find the measure of angle z in the following figure
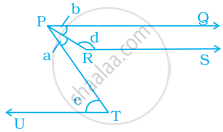
Find the value of angle a in the following figure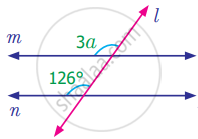
Find the value of angle a in the following figure
Find the value of angle x in the following figure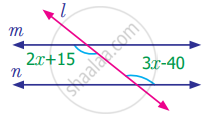
Find the value of z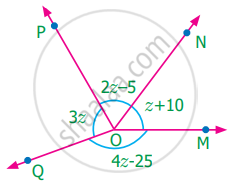
In the figure, the lines GH and IJ are parallel. If ∠1 = 108° and ∠2 = 123°, find the value of x, y and z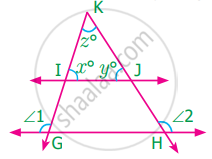
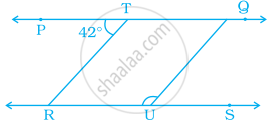
In the given figure, PQ || RS, TR || QU and ∠PTR = 42°. Find ∠QUR.
In the given figure, PQ, RS and UT are parallel lines. If c = 57° and a = `c/3`, find the value of d.