Advertisements
Advertisements
Question
In the given figure, line DE || line GF, ray EG and ray FG are bisectors of ∠DEF and ∠DFM respectively. Prove that,
- ∠DEG = `1/2∠"EDF"`
- EF = FG
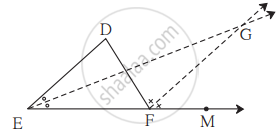
Solution
(i) ∠DEG = ∠FEG = x° ...(i)[Ray EG bisects ∠DEF]
∠GFD = ∠GFM = y° ...(ii)[Ray FG bisects ∠DFM]
line DE || line GF and DF is their transversal. ...[Given]
∴ ∠EDF = ∠GFD ...[Alternate angles]
∴ ∠EDF = y° ...(iii) [From (ii)]
line DE || line GF and EM is their transversal. ...[Given]
∴ ∠DEF = ∠GFM ...[Corresponding angles]
∴ ∠DEG + ∠FEG = ∠GFM ...[Angle addition property]
∴ x° + x° = y° ...[From (i) and (ii)]
∴ 2x° = y°
∴ x° = `1/2`y°
∴ ∠DEG = `1/2`∠EDF ...[From (i) and (iii)]
(ii) line DE || line GF and GE is their transversal. ...[Given]
∴ ∠DEG = ∠FGE ...(iv)[Alternate angles]
∴ ∠FEG = x° ...(v)[From (i) and (iv)]
∴ In ∆FEG,
∠FEG = ∠FGE ...[From (v)]
∴ EF = FG ...[Converse of isosceles triangle theorem]
APPEARS IN
RELATED QUESTIONS
In the given figure, ∠ACD is an exterior angle of ΔABC. ∠B = 40°, ∠A = 70°. Find the measure of ∠ACD.

In ΔPQR, ∠P = 70°, ∠Q = 65° then find ∠R.
The measures of angles of a triangle are x°, (x - 20)°, (x - 40)°. Find the measure of each angle.
The measure of one of the angles of a triangle is twice the measure of its smallest angle and the measure of the other is thrice the measure of the smallest angle. Find the measures of the three angles.
In the given figure, measures of some angles are given. Using the measures find the values of x, y, z.

In the given figure, line AB || line DE. Find the measures of ∠DRE and ∠ARE using given measures of some angles.

In Δ ABC, bisectors of ∠A and ∠B intersect at point O. If ∠C = 70°. Find the measure of ∠AOB.
In the given Figure, line AB || line CD and line PQ is the transversal. Ray PT and ray QT are bisectors of ∠BPQ and ∠PQD respectively. Prove that m∠PTQ = 90°.

Using the information shown in figure, find the measures of ∠a, ∠b and ∠c.

