Advertisements
Advertisements
Question
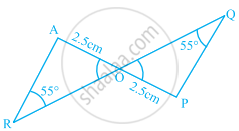
In the given pairs of triangles of figure, applying only ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.
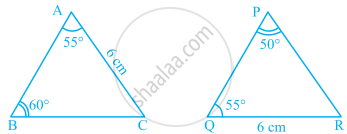
Solution
In the given figure, ∆ABC and ∆POR are not congruent.
APPEARS IN
RELATED QUESTIONS
Given below are measurements of some parts of two triangles. Examine whether the two triangles are congruent or not, by the ASA congruence rule. In the case of congruence, write it in symbolic form.
∆DEF, ∠D = 60º, ∠F = 80º, DF = 6 cm.
∆PQR, ∠Q = 60º, ∠R = 80º, QP = 6 cm.
In the given figure, AC ≡ AD and ∠CBD ≡ ∠DEC. Prove that ∆BCF ≡ ∆EDF.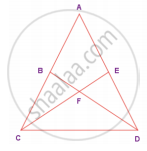
In the figure, ∠TMA ≡∠IAM and ∠TAM ≡ ∠IMA. P is the midpoint of MI and N is the midpoint of AI. Prove that ΔPIN ~ ΔATM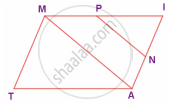
State whether the two triangles are congruent or not. Justify your answer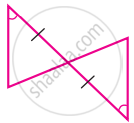
To conclude the congruency of triangles, mark the required information in the following figure with reference to the given congruency criterion
Two triangles are congruent, if two angles and the side included between them in one of the triangles are equal to the two angles and the side included between them of the other triangle. This is known as the ______.
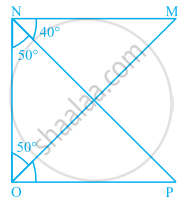
In the given figure, ∆ARO ≅ ∆______.
In the given figure, AD ⊥ BC and AD is the bisector of angle BAC. Then, ∆ABD ≅ ∆ACD by RHS.

In the given pairs of triangles of figure, applying only ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.

In the given pairs of triangles of figure, applying only ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.