Advertisements
Advertisements
Question
In the given figure, AC ≡ AD and ∠CBD ≡ ∠DEC. Prove that ∆BCF ≡ ∆EDF.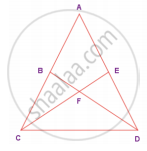
Solution
| Statements | Reasons |
| 1. ∠BCF = ∠EFD | Vertically opposite angles |
| 2. ∠CBD = ∠DEC | Angles on the same base given |
| 3. ∠BCF = ∠EDF | Remaining angles of ∆BCF and ∆EDF |
| 4. ∆BCF ≡ ∆EDF | By (1) and (2) AAA criteria |
APPEARS IN
RELATED QUESTIONS
By applying the ASA congruence rule, it is to be established that ∆ABC ≅ ∆QRP and it is given that BC = RP. What additional information is needed to establish the congruence?
In Fig, can you use the ASA congruence rule and conclude that ∆AOC ≅ ∆BOD?

Given below are measurements of some parts of two triangles. Examine whether the two triangles are congruent or not, by the ASA congruence rule. In the case of congruence, write it in symbolic form.
∆DEF, ∠D = 60º, ∠F = 80º, DF = 6 cm.
∆PQR, ∠Q = 60º, ∠R = 80º, QP = 6 cm.
Consider the given pairs of triangles and say whether each pair is that of congruent triangles. If the triangles are congruent, say ‘how’; if they are not congruent say ‘why’ and also say if a small modification would make them congruent:
ΔABC and ΔDEF are two triangles in which AB = DF, ∠ACB = 70°, ∠ABC = 60°, ∠DEF = 70° and ∠EDF = 60°. Prove that the triangles are congruent
State whether the two triangles are congruent or not. Justify your answer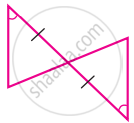
For the given pair of triangles state the criterion that can be used to determine the congruency?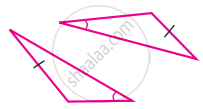
AAS congruence criterion is same as ASA congruence criterion.
In the given pairs of triangles of figure, applying only ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.
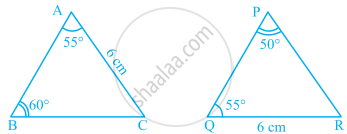
In the given pairs of triangles of figure, applying only ASA congruence criterion, determine which triangles are congruent. Also, write the congruent triangles in symbolic form.

