Advertisements
Advertisements
Question
In the given figure, ΔBCD is isosceles with base BD and ∠BAE ≡∠DEA. Prove that AB ≡ ED
Solution
| Statements | Reasons |
| 1. ∠BAE ≡ ∠DEA | Given |
| 2. AC = EC | By (1) sides opposite to equal angles are equal |
| 3. BC = DC | Given BCD is isosceles with base BD |
| 4. AC – BC = EC – DC | 2 – 3 |
| 5. AB ≡ ED | By 4 |
APPEARS IN
RELATED QUESTIONS
State whether the two triangles are congruent or not. Justify your answer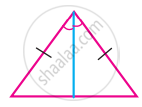
For the given pair of triangles state the criterion that can be used to determine the congruency?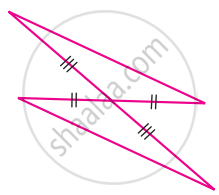
Construct a triangle XYZ with the given conditions.
BC = 8 cm, AC = 6 cm and ∠C = 40°
Construct a triangle PQR with given conditions.
∠P = 60°, ∠R = 35° and PR = 7.8 cm
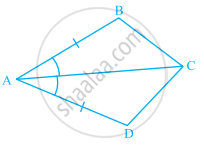
In the given figure, AB = AD and ∠BAC = ∠DAC. Then
- ∆ ______ ≅ ∆ABC.
- BC = ______.
- ∠BCA = ______.
- Line segment AC bisects ______ and ______.
In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.

In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.

In the given figure, which pairs of triangles are congruent by SAS congruence criterion (condition)? if congruent, write the congruence of the two triangles in symbolic form.
State which of the following pairs of triangles are congruent. If yes, write them in symbolic form (you may draw a rough figure).
∆ABC: AB = 4.8 cm, ∠A = 90°, AC = 6.8 cm
∆XYZ: YZ = 6.8 cm, ∠X = 90°, ZX = 4.8 cm
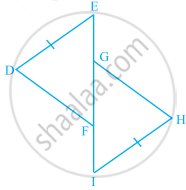
In the given figure, DE = IH, EG = FI and ∠E = ∠I. Is ∆DEF ≅ ∆HIG? If yes, by which congruence criterion?