Advertisements
Advertisements
Question
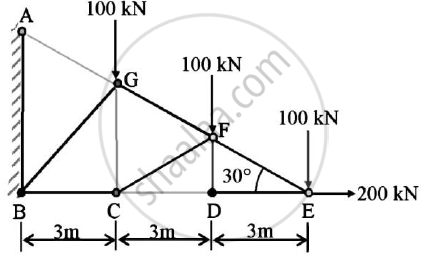
In the truss shown in figure,compute the forces in each member.

Solution
Solution :
We can say that FD,GH and CB are zero force members in the given truss
Joint A :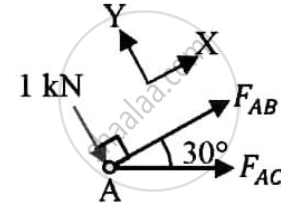
Applying the conditions of equilibrium
ΣFy=0
-1 – FAC sin30 = 0
FAC = -2kN
Applying the conditions of equilibrium
ΣFx = 0
FAB + FAC cos30 = 0
FAB = 1.7321 Kn
JOINT C :
Applying the conditions of equilibrium
ΣFx = 0
FCE = FCA = -2kN
JOINT B :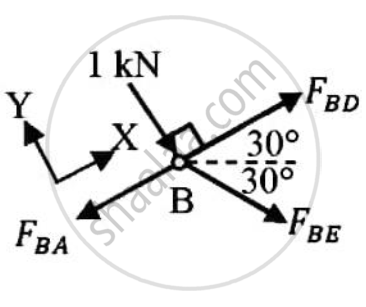
Applying the conditions of equilibrium
ΣFy = 0
-1 - FBE sin60 = 0
FBE = -1.1547 kN
Applying the conditions of equilibrium
ΣFx = 0
-FBA + FBEcos60 + FBD = 0
FBD = 2.3094 kN
JOINT D :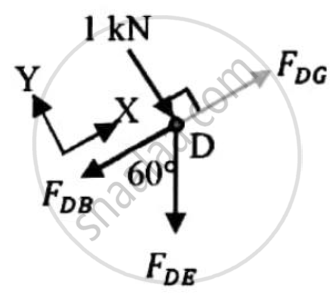
Applying the conditions of equilibrium
ΣFy = 0
-1 - FDEsin60 = 0
FDE = -1.1547 kN
Applying the conditions of equilibrium
ΣFx = 0
-FDB - FDEcos60 + FDG = 0
FDG = 1.7321 kN
JOINT E :
Applying the conditions of equilibrium
ΣFy = 0
FED + FEFcos30 + FEBsin30 = 0
FEFcos30 = -(-1.1547)-(-1.1547) x`1/2`
FEF = 2kN
Joint F :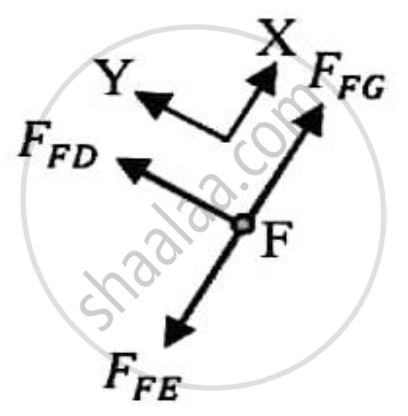
Applying the conditions of equilibrium
ΣFx = 0
FFG = FFE = -2kN
Final answer :
| Sr.no. | MEMBER | MAGNITUDE OF FORCE (in kN) | NATURE OF FORCE |
| 1. | AC | 2 | COMPRESSION |
| 2. | AB | 1.7321 | TENSION |
| 3. | CB | 0 | - |
| 4. | CE | 2 | COMPRESSION |
| 5. | BE | 1.1547 | COMPRESSION |
| 6. | BD | 2.3094 | TENSION |
| 7. | DE | 1.1547 | COMPRESSION |
| 8.. | DG | 1.7321 | TENSION |
| 9. | EF | 2 | TENSION |
| 10. | EH | 4 | COMPRESSION |
| 11. | FD | 0 | - |
| 12. | FG | 2 | COMPRESSION |
| 13. | GH | 0 | - |
APPEARS IN
RELATED QUESTIONS
Referring to the truss shown in the figure. Find :
(a) Reaction at D and C
(b)Zero force members.
(c)Forces in member FE and DC by method of section.
(d)Forces in other members by method of joints.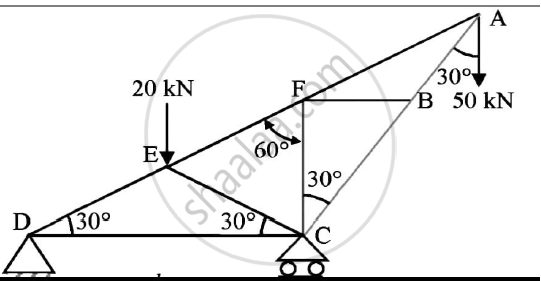
What is a zero force member in a truss? With examples state the conditions for a zero force member.
A truss is loaded and supported as shown.Determine the following:
(1)Identify the zero force members,if any
(2)Find the forces in members EF,ED and FC by method of joints.
(3)Find the forces in members GF,GC and BC by method of sections