Advertisements
Advertisements
Question

Point G is the centroid of ABC.
If l(RG) = 2.5 then l(GC) = ______.
Solution
If l(RG) = 2.5 then l(GC) = 5.
Explanation:
In ∆ABC, the medians AP, BQ, and CR to the sides BC, CA, and AB respectively intersect at G. Since then the centroid of a triangle divides the medians in the ratio of 2 : 1, then AG : GP = BG :
GQ = CG : GR = 2 : 1
We have, CG: GR = 2 : 1
⇒ `("GC")/("RG") = 2/1`
⇒ `("GC")/(2.5) = 2/1`
⇒ GC = 5
RELATED QUESTIONS
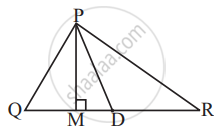
In ΔPQR, D is the mid-point of `bar(QR)`.
`bar(PM)` is ______.
PD is ______.
Is QM = MR?
Draw rough sketch for the following:
In ΔPQR, PQ and PR are altitudes of the triangle.
Draw rough sketch for the following:
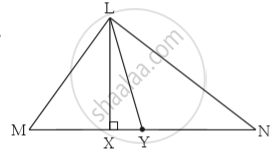
In ΔXYZ, YL is an altitude in the exterior of the triangle.
In Δ LMN, _____ is an altitude and _____ is a median. (write the names of appropriate segments.)
Draw an acute angled Δ PQR. Draw all of its altitudes. Name the point of concurrence as ‘O’.
Name the orthocentre of ∆PQR
The triangle ABC formed by AB = 5 cm, BC = 8 cm, AC = 4 cm is ______.
How many altitudes does a triangle have?
Which of the following figures will have it’s altitude outside the triangle?
The ______ triangle always has altitude outside itself.
