Question

Prove that the perimeter of a triangle is greater than the sum of its altitudes. 

Answer 1

Given: A ΔABC in which , AD ⊥BC ,BE ⊥ AC  and . CF ⊥ AB .
To prove: 

AD+BE+CF+<AB+BC+AC 

Figure: 

  

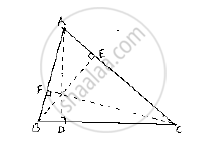
Proof:
We know that of all the segments that can be drawn to a given line, from a point not lying on it, the perpendicular distance i.e., the perpendicular line segment is the shortest.
Therefore, 

 AD⊥ BC 

⇒AB>AC and AC> AD 

⇒AB+AC>2AD         ............(1) 

Similarly BE ⊥ AC 

⇒BA>BE and BC>BE   

⇒BA+BC>2BE .............(2) 

And also CF⊥ AB 

⇒CA> CF and CB>CF 

⇒ CA+CB>2CF          ..............(3) 

Adding (1), (2) and (3), we get 

AB+AC+BA+BC+CA+CB>2AD+2BE+2CF 

⇒2AB+2BC+2CA>2(AD+BE+CF) 

⇒2(AB+BC+CA)>2(AD+BE+CF) 

⇒AB+BC+CA>AD+BE+CF  

⇒The perimeter of the triangle is greater than the sum of its altitudes
∴ Hence proved