Advertisements
Advertisements
Question
Put three different numbers in the circles so that when you add the numbers at the end of each line you always get a perfect square.
Solution
6, 19 and 30 are the three numbers in which, when we add the end of each line we always get a perfect square.
6 + 19 = 25
6 + 30 = 36
19 + 30 = 49 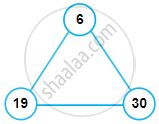
APPEARS IN
RELATED QUESTIONS
Find the square of the given number.
35
Find the square of the given number.
86
Find the square of the given number.
46
What will be the units digit of the square of the following number?
52
Which of the following triplet pythagorean?
(10, 24, 26)
Observe the following pattern \[1 = \frac{1}{2}\left\{ 1 \times \left( 1 + 1 \right) \right\}\]
\[ 1 + 2 = \frac{1}{2}\left\{ 2 \times \left( 2 + 1 \right) \right\}\]
\[ 1 + 2 + 3 = \frac{1}{2}\left\{ 3 \times \left( 3 + 1 \right) \right\}\]
\[1 + 2 + 3 + 4 = \frac{1}{2}\left\{ 4 \times \left( 4 + 1 \right) \right\}\]and find the values of following:
31 + 32 + ... + 50
The sum of successive odd numbers 1, 3, 5, 7, 9, 11, 13 and 15 is ______.
The dimensions of a rectangular field are 80 m and 18 m. Find the length of its diagonal.
A 5.5 m long ladder is leaned against a wall. The ladder reaches the wall to a height of 4.4 m. Find the distance between the wall and the foot of the ladder.
