Question

Show that the following equations: -2x + y + z = a, x - 2y + z = b, x + y - 2z = c have no solutions unless a +b + c = 0 in which case they have infinitely many solutions. Find these solutions when a=1, b=1, c=-2. 

Answer 1

Part I:
-2x + y + z = a
x - 2y + z = b
x + y - 2z = c
Writing the equations in the matrix form, 

`[[-2,1,1],[1,-2,1],[1,1,-2]].[[x],[y],[z]]=[[a],[b],[a+b+c]]`

Augmented matrix [A|B]=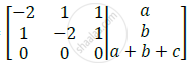

Number of unknowns = n = 3
Rank of A (rA) = Number of non-zero rows in A = 2

Case I: No Solution
For which, rA < rAB
This is only possible, when ‘a+b+c≠0’ upon which,
Rank of [A|B] = (rAB) = 3 

Case II: Infinite Solution
For which, rA = rAB < n (i.e. < 3)
This is only possible, when ‘a+b+c=0’ upon which,
Rank of [A|B] = rAB = 2 

Part II: Put a= 1, b = 1, c = -2, in (1) 

`[[-2,1,1],[1,-2,1],[0,0,0]].[[x],[y],[z]]=[[1],[1],[0]]`

`R^2 – R^1;` → `[[-2,1,1],[3,-3,0],[0,0,0]].[[x],[y],[z]]=[[1],[0],[0]]`                        …(2)

Here, n – rA = 3 – 2 = 1
We have to assume one unknown.
Let y = t (≠0)
On expanding (2), 3x – 3y = 0
∴ x – y = 0
∴ x = y = t
And, -2x + y + z = 1
∴ -2t + t + z = 1
∴z = 1 + t
Hence, the solution is x = t, y = t, z = 1 + t (Infinite Solution)