Advertisements
Advertisements
Question
Solve the following L.P.P. graphically:
Maximize Z = 60x + 40y
Subject to x + 2y ≤ 12
2x + y ≤ 12
4x + 5y ≥ 20
x, y ≥ 0
Sum
Solution
Given LPP is
Max Z = 60x + 40y
Subject to x + 2y ≤ 12 ...(i)
2x + y ≤ 12 ...(ii)
4x + 5y ≥ 20 ...(iii)
x, y ≥ 0
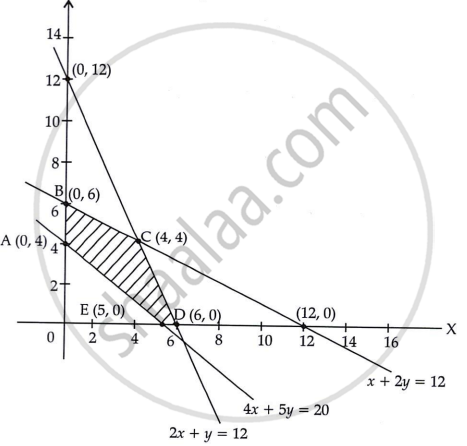
| Corner points | Z = 60x + 40y |
| A(0, 4) | 1600 |
| B(0, 6) | 2400 |
| C(4, 4) | 4000 (max) |
| D(6, 0) | 3600 |
| E(5, 0) | 3000 |
Thus, maximum value of Z is 4000 which occurs at the point c (4, 4).
shaalaa.com
Is there an error in this question or solution?
