Advertisements
Advertisements
Question
State Einstein’s photoelectric equation. Explain all characteristics of the photoelectric effect, on the basis of Einstein’s photoelectric equation.
Solution
Einstein’s photoelectric equation: K.E.max = (hν – `phi_0`)
Characteristics of photoelectric effect:
- The photoelectric work function `phi_0` is constant for a given emitter. Hence if the frequency ‘ν’ of the incident radiation is decreased, the maximum kinetic energy of the emitted photoelectrons decreases, till it becomes zero for a certain frequency ν0. Therefore, from Einstein’s equation,
0 = hv0 - `phi_0`
∴ `phi_0` = hv0 .......….(1)
This shows that the threshold frequency is related to the work function of the metal and hence it has different values for different metals. - Using equation (1), Einstein’s equation can be written as
`1/2"mv"_"max"^2 = "hv" - "hv"_0`
∴ `1/2"mv"_"max"^2 = "h"("v" - "v"_0)`
This equation shows that:
a. If ν < ν0, then K.E is negative, which is not possible. In this case, photoelectric emission is not possible.
b. If ν > ν0, then photoelectrons move with some velocity.
∴ K.E > 0, which is possible. Hence, photoelectrons are emitted.
c. If ν = ν0, the photoelectrons are just emitted. In this case, K.E = 0. - The photoelectric equation is,
`1/2"mv"_"max"^2 = "hv" - phi_0` .....….(2)
where, hν = energy of the photon of incident radiation.
`phi_0 = "hv"_0` = photoelectric work function of the metal.
Thus, both the terms on the R.H.S of equation (2) depends on the frequency and not on the intensity of radiation. Hence the maximum kinetic energy with which photoelectrons are emitted is independent of the intensity of radiation. However, since `phi_0` and h are constants, the maximum kinetic energy of the photoelectrons is directly proportional to the frequency. - According to the quantum theory, when the intensity of the radiation increases, there is a proportional increase in the number of photons incident per second on the surface. One photon can cause the emission of one photoelectron. Therefore, with the increase in the intensity of radiation, there will be an increase in the photoelectron interactions and the rate of emission of electrons.
- The emission of a photoelectron is the result of a collision between a photon and an electron. As soon as the radiation is incident on the photosensitive surface, the entire energy of the photon is absorbed by the electron at once. Therefore, the electrons are emitted at a moment when light is incident on the metal surface. This explains why photoelectric emission is instantaneous.
- If the electron, with which the incident photon collides, is situated on the emitting surface, the electron will be ejected with maximum K.E as given by Einstein’s equation.
- If, however, the electron is situated in the interior of the emitting material, it will lose some energy in coming to the surface. This explains why the photoelectrons are emitted with different kinetic energies.
Thus, all the features of the photoelectric effect are explained.
APPEARS IN
RELATED QUESTIONS
Is it always possible to see the photoelectric effect with a red light?
Using the values of work function given in the following table, tell which metal will require the highest frequency of incident radiation to generate photocurrent.
Typical values of work function for some common metals
| Metal | Work function (in eV) |
| Potassium | 2.3 |
| Sodium | 2.4 |
| Calcium | 2.9 |
| Zinc | 3.6 |
| Silver | 4.3 |
| Aluminium | 4.3 |
| Tungsten | 4.5 |
| Copper | 4.7 |
| Nickel | 5.0 |
| Gold | 5.1 |
Given the following data for incident wavelength and the stopping potential obtained from an experiment on the photoelectric effect, estimate the value of Planck's constant and the work function of the cathode material. What is the threshold frequency and corresponding wavelength? What is the most likely metal used for emitter?
| Incident wavelength (in Å) | 2536 | 3650 |
| Stopping potential (in V) |
1.95 | 0.5 |
If the total energy of radiation of frequency 1014 Hz is 6.63 J, Calculate the number of photons in the radiation.
State Einstein photoelectric equation. Explain 2 characteristics of the photoelectric effect on the basis of Einstein’s photoelectric equation.
The maximum velocity of photoelectron emitted is 4.8 m/s. If the e/m ratio of the electron is 1.76 × 1011 C/kg, then stopping potential is given by ______
The ratio of energies of photons produced due to transition of electron of hydrogen atom from its (i) second to first energy level and (ii) highest energy level to second level is respectively.
If the maximum kinetic energy of emitted electrons in photoelectric effect is 3.2 × 10-19 J and the work-function for metal is 6.63 × 10-19 J, then stopping potential and threshold wavelength respectively are
[Planck's constant, h = 6.63 × 1034 J-s]
[Velocity of light, c = 3 × 108 `"m"/"s"`]
[Charge on electron= 1.6 × 10-19 C]
For photoelectric emission from certain metal, the cut-off frequency is v. If radiation of frequency 2v impinges on the metal plate, the maximum possible velocity of the emitted electron will be (m is the electron mass) ____________.
Threshold frequency for a metal is 1015 Hz. Light of `lambda` = 4000 Å falls on its surface. Which of the following statements is correct?
In photoelectric effect, for a light of different intensities but of same frequency, the stopping potential for a given metal is ____________.
A metal surface is illuminated by photons of energy 5 eV and 2.5 eV respectively. The ratio of their wavelengths is ____________.
When a surface 1 cm thick is illuminated by light of wavelength 'λ', the stopping potential is 'V0'. When the same surface is illuminated by light of wavelength '3λ', the stopping potential is `"V"_0/6`. The threshold wavelength for the metallic surface is ______.
Following graphs show the variation of stopping potential corresponding to the frequency of incident radiation (F) for a given metal. The correct variation is shown in graph (v0 = Threshold frequency).
The photon of frequency vis incident on a metal surface whose threshold frequency is v0. The kinetic energy of the emitted photoelectrons will be ______.
A metal surface having work function 'w0' emits photoelectrons when photons of energy 'E' are incident on it. The electron enters the uniform magnetic field (B) in perpendicular direction and moves in circular path of radius 'r'. Then 'r' is equal to (m and e be the mass and charge of electron respectively) ____________.
The ratio of slopes m1: ro2 of the lines given in the following graphs is, ______.
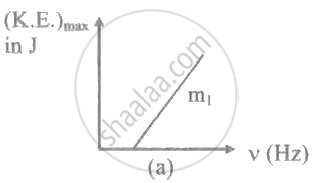
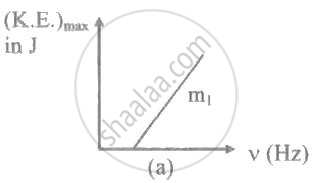
When the work function of a metal increases, maximum kinetic energy of emitted photoelectrons ____________.
The radiation corresponding to the 3 → 2 transition of a hydrogen atom falls on a gold surface to generate photoelectrons. These electrons are passed through a magnetic field of 5 × 10-4 T. Assume that the radius of the largest circular path followed by these electrons is 7 mm, and the work function of the metal is ______.
(Mass of electron = 9.1 × 10-31 kg)
The radiation emitted, when an electron jumps from n = 3 to n = 2 orbit is a hydrogen atom, falls on a metal to produce photoelectron. The electrons from the metal surface with maximum kinetic energy are made to move perpendicular to a magnetic field of `1/320`T in a radius of 10-3m. Find the 320 work function of metal:
A charged dust particle of radius 5 × 10-7 m is located in a horizontal electric field having an intensity of 6.28 × 105 V/m. The surrounding medium is air with a coefficient of viscosity η = 1.6 × 10-5 N-s/m2. If the particle moves with a uniform horizontal speed of 0.02 m/s, the number of electrons on it is ______.
Photoelectric emission is observed from a metallic surface for frequencies ν1 and ν2 of the incident light rays (ν1 > ν2). If the ratio of the maximum value of the kinetic energy of the photoelectrons emitted in the first case to that in the second case is 2 : K, then the threshold frequency of the metallic surface is ______.
If the maximum kinetic energy of emitted electrons in the photoelectric effect is 2eV, the stopping potential will be ______.
Explain the failure of wave theory of light to account for the observations from experiments on photoelectric effect.
By increasing the voltage in an electron diffraction tube, the radius of the diffraction rings will ______.
Explain the formation of clouds at high altitude.
In a photoelectric experiment, the stopping potential is 1.5V. What is the maximum kinetic energy of a photoelectron?
