Advertisements
Advertisements
Question
Suppose the rod in the previous problem has a mass of 1 kg distributed uniformly over its length.
(a) Find the initial angular acceleration of the rod.
(b) Find the tension in the supports to the blocks of mass 2 kg and 5 kg.
Solution
Total moment of inertia of the system about the axis of rotation,
\[I_{net} = \left( m_1 r_1^2 + m_2 r_2^2 + \frac{m l^2}{12} \right)\]
m and l are the mass and length of the rod, respectively.
\[ \tau_{net} = F_1 r_1 - F_2 r_2 \]
\[\text{Also, }\tau_{net} = I_{net} \times \alpha\]
On equating the value of \[\tau_{net}\] and putting the value of `l_("net"),` we get
\[F_1 r_1 - F_2 r_2 = \left( m_1 r_1^2 + m_2 r_2^2 + \frac{m l^2}{12} \right) \times \alpha\]
\[\left( - 2 \times 10 \times 0 . 5 \right) + \left( 5 \times 10 \times 0 . 5 \right) = \left[ 5 \left( \frac{1}{2} \right)^2 + 2 \left( \frac{1}{2} \right)^2 + \frac{\left( 1 \right)^2}{12} \right] \alpha\]
\[\Rightarrow 15 = \left( 1 . 75 + 0 . 084 \right) \alpha\]
\[ \Rightarrow \alpha = \frac{1500}{\left( 175 + 8 . 4 \right)} = \frac{1500}{183 . 4}\]
\[= 8 . 1\text{ rad/s}^2 ........\left( g = 10 \right)\]
\[= 8 . 01\text{ rad/s}^2 ......\left(\text{if }g = 9 . 8 \right)\]
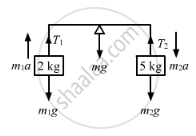
(b) From the free body diagram of the block of mass 2 kg,
\[T_1 - m_1 g = m_1 a\]
\[ \Rightarrow T_1 = 2 \left( a + g \right)\]
\[ = 2\left( \alpha r + g \right)........ \left(\text{using, }a = \alpha r \right)\]
\[ = 2\left( 8 \times 0 . 5 + 9 . 8 \right)\]
\[ \Rightarrow T_1 = 27 . 6 N\]
From the free body diagram of the block of mass 5 kg,
\[m_2 g - T_2 = m_2 a\]
\[ \Rightarrow T_2 = m_2 \left( g - a \right)\]
\[ = 5 \left( g - a \right) = 5 \left( 9 . 8 - 8 \times 0 . 5 \right)............\left( a = \alpha r \right)\]
\[= 5 \times 5 . 8 = 29 N\]
APPEARS IN
RELATED QUESTIONS
A cubical block of mass M and edge a slides down a rough inclined plane of inclination θ with a uniform velocity. The torque of the normal force on the block about its centre has a magnitude
A solid sphere, a hollow sphere and a disc, all having same mass and radius, are placed at the top of a smooth incline and released. Least time will be taken in reaching the bottom by _________ .
A string of negligible thickness is wrapped several times around a cylinder kept on a rough horizontal surface. A man standing at a distance l from the cylinder holds one end of the string and pulls the cylinder towards him (see the following figure). There is no slipping anywhere. The length of the string passed through the hand of the man while the cylinder reaches his hands is _________ .
Consider a wheel of a bicycle rolling on a level road at a linear speed \[\nu_0\] (see the following figure)
(a) the speed of the particle A is zero
(b) the speed of B, C and D are all equal to \[v_0\]
(c) the speed of C is 2 \[v_0\]
(d) the speed of B is greater than the speed of O.

Three particles, each of mass 200 g, are kept at the corners of an equilateral triangle of side 10 cm. Find the moment of inertial of the system about an axis joining two of the particles.

Three particles, each of mass 200 g, are kept at the corners of an equilateral triangle of side 10 cm. Find the moment of inertial of the system about an axis passing through one of the particles and perpendicular to the plane of the particles.

Particles of masses 1 g, 2 g, 3 g, .........., 100 g are kept at the marks 1 cm, 2 cm, 3 cm, ..........., 100 cm respectively on a metre scale. Find the moment of inertia of the system of particles about a perpendicular bisector of the metre scale.
The radius of gyration of a uniform disc about a line perpendicular to the disc equals its radius. Find the distance of the line from the centre.
Find the moment of inertia of a uniform square plate of mass m and edge a about one of its diagonals.
The surface density (mass/area) of a circular disc of radius a depends on the distance from the centre as [rholeft( r right) = A + Br.] Find its moment of inertia about the line perpendicular to the plane of the disc thorough its centre.
Because of the friction between the water in oceans with the earth's surface the rotational kinetic energy of the earth is continuously decreasing. If the earth's angular speed decreases by 0⋅0016 rad/day in 100 years find the average torque of the friction on the earth. Radius of the earth is 6400 km and its mass is 6⋅0 × 1024 kg.
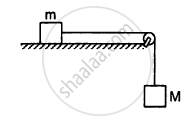
The following figure shows two blocks of mass m and M connected by a string passing over a pulley. The horizontal table over which the mass m slides is smooth. The pulley has a radius r and moment of inertia I about its axis and it can freely rotate about this axis. Find the acceleration of the mass M assuming that the string does not slip on the pulley.
A sphere of mass m rolls on a plane surface. Find its kinetic energy at an instant when its centre moves with speed \[\nu.\]
A small spherical ball is released from a point at a height h on a rough track shown in the following figure. Assuming that it does not slip anywhere, find its linear speed when it rolls on the horizontal part of the track.

