Advertisements
Advertisements
Question
The acceleration of a particle is zero, as measured from an inertial frame of reference. Can we conclude that no force acts on the particle?
Solution
No. The acceleration of the particle can also be zero if the vector sum of all the forces is zero, i.e. no net force acts on the particle.
APPEARS IN
RELATED QUESTIONS
Define one newton force.
Is it possible for a particle to describe a curved path if no force acts on it? Does your answer depend on the frame of reference chosen to view the particle?
Consider a book lying on a table. The weight of the book and the normal force by the table in the book are equal in magnitude and opposite in direction. Is this an example of Newton's third law?
A plumb bob is hung from the ceiling of a train compartment. If the train moves with an acceleration 'a' along a straight horizontal track , the string supporting the bob makes an angle tan−1 (a/g) with the normal to the ceiling. Suppose the train moves on an inclined straight track with uniform velocity. If the angle of incline is tan−1 (a/g), the string again makes the same angle with the normal to the ceiling. Can a person sitting inside the compartment tell by looking at the plumb line whether the train is accelerating on a horizontal straight track or moving on an incline? If yes, how? If not, then suggest a method to do so.
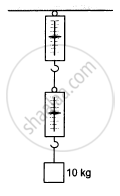
A block of mass 10 kg is suspended from two light spring balances, as shown in the following figure.
A reference frame attached to the earth
(a) is an inertial frame by definition
(b) cannot be an inertial frame because the earth is revolving around the sun
(c) is an inertial frame because Newton's laws are applicable in this frame
(d) cannot be an inertial frame because the earth is rotating about its axis.
A particle stays at rest as seen in a frame. We can conclude that
(a) the frame is inertial
(b) resultant force on the particle is zero
(c) the frame may be inertial but the resultant force on the particle is zero
(d) the frame may be non-inertial but there is a non-zero resultant force
A particle is found to be at rest when seen from a frame S1 and moving with constant velocity when seen from another frame S2. Mark out the possible options.
(a) Both the frames are inertial.
(b) Both the frames are non-inertial.
(c) S1 is inertial and S2 is non-inertial.
(d) S1 is non-inertial and S2 is inertial
A particle is observed from two frames S1 and S2. Frame S2 moves with respect to S1with an acceleration a. Let F1 and F2 be the pseudo forces on the particle when seen from S1 and S2, respectively. Which of the following is not possible?
'When a hanging carpet is beaten with a stick, the dust particles start coming out of it'. This phenomenon can be best explained by making use of :
The greater is the __________ the greater is the inertia of an object.
Differentiate between gravitational mass and inertial mass.
If a 5 N and a 15 N forces are acting opposite to one another. Find the resultant force and the direction of action of the resultant force.
Newton's first law of motion describes ______.
A car of mass m starts from rest and acquires a velocity along east `v = vhati (v > 0)` in two seconds. Assuming the car moves with uniform acceleration, the force exerted on the car is ______.
In the above given problem if the lower thread is pulled with a jerk, what happens?
A smooth sphere of radius R and mass M is placed on the smooth horizontal floor. Another smooth particle of mass m is placed on the sphere and a horizontal force F is applied on the sphere as shown. If the particle does not slip on the sphere then the value of force F is ______.

A balloon has mass of 10 g in air. The air escapes from the balloon at a uniform rate with velocity 4.5 cm/s. If the balloon shrinks in 5 s completely. Then, the average force acting on that balloon will be (in dyne).
This question has Statement 1 and Statement 2. Of the four choices given after the Statements, choose the one that best describes the two Statements.
Statement 1: If you push on a cart being pulled by a horse so that it does not move, the cart pushes you back with an equal and opposite force.
Statement 2: The cart does not move because the force described in statement 1 cancel each other.
