Question

The area bounded by ${\displaystyle y}$-axis, ${\displaystyle y=\cos x}$ and ${\displaystyle y=\sin x,0 \le x-\frac{<\pi }{2}}$ is

Options

• ${\displaystyle 2(\sqrt{2}-1)}$

• ${\displaystyle \sqrt{2}-1}$

• ${\displaystyle \sqrt{2}+1}$

• ${\displaystyle \sqrt{2}}$

Answer 1

${\displaystyle \sqrt{2}-1}$

Explanation:

The curves are ${\displaystyle y=\cos x,y=\sin x,0 \le x-\frac{<\pi }{2}}$

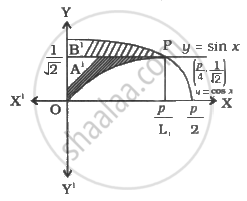
The curves meet where ${\displaystyle \sin x=\cos x}$ to ${\displaystyle \tan x=1}$

⇒ ${\displaystyle x=\frac{\pi }{2},\sin \frac{\pi }{4}=\cos \frac{\pi }{4}=\frac{1}{\sqrt{2}}}$

Graphs of these curve is as shown in figure.

They intersect at ${\displaystyle P(\frac{\pi }{4},\frac{1}{\sqrt{2}})}$

The area bounded by ${\displaystyle y}$-axis, ${\displaystyle y=\cos x}$ and ${\displaystyle y=\sin x(0\le x\frac{<\pi }{2})}$

= Shaded area = Area of region ΔOPBO

= Area of region ΔPAO + Area of region ΔPBA

= ${\displaystyle {\int }_0^{\frac{1}{\sqrt{2}}}{\sin }^{-1} ydy+{\int }_{\frac{1}{\sqrt{2}}}^1{\cos }^{-1} ydy}$

= Integrating each by parts taking II as first function

A = ${\displaystyle {[y{\sin }^{-1} y-\int \frac{1}{\sqrt{1-y^2}}\cdot ydy]}_0^{\frac{1}{\sqrt{2}}}+{[y{\cos }^{-1}y+ \int \frac{1}{\sqrt{1-y^2}}ydy]}_{\frac{1}{\sqrt{2}}}^1}$

= ${\displaystyle {[y{\sin }^{-1} y+\sqrt{1-y^2}]}_0^{\frac{1}{\sqrt{2}}}+{[y{\cos }^{-1}y-\sqrt{1-y^2}]}_{\frac{1}{\sqrt{2}}}^1}$

= ${\displaystyle [\frac{1}{\sqrt{2}}{\sin }^{-1} \frac{1}{\sqrt{2}}+\sqrt{\frac{1}{2}}-1]+[\left({\cos }^{-1}-0\right)-(\frac{1}{\sqrt{2}}{\cos }^{-1} \frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}})]}$

= ${\displaystyle (\frac{1}{\sqrt{2}}\cdot \frac{\pi }{4}+\frac{1}{\sqrt{2}})+(0-\frac{1}{\sqrt{2}}\cdot \frac{\pi }{4}+\frac{1}{\sqrt{2}})}$

= ${\displaystyle 2\times \frac{1}{\sqrt{2}}-1}$

= ${\displaystyle \sqrt{2}-1}$.