Advertisements
Advertisements
Question
The average cost function, AC for a commodity is given by AC = `x + 5 + 36/x` in terms of output x. Find
1) The total cost, C and marginal cost, MC as a function of x.
2) The outputs for which AC increases
Solution
Let C be the total cost function. Then
Average cost (AC) = `C/x => AC.x => C = (x + 5 + 36/x) x`
`=> C = x^2 + 5x + 36`
Let MC be the marginal cost function. Then,
`MC = (dC)/dx = d/dx (x^2 + 5x + 36) = 2x + 5`
Now, `d/dx (AC) = d/dx (x + 5 + 36/x) = 1 - 36/x^2`
For AC to be increasing `d/dx(AC) > 0 => 1 - 36/x^2 > 0`
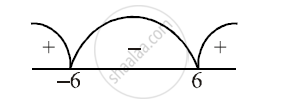
`=> x^2 - 36 > 0 => (x - 6) (x + 6) > 0`
`=> x < -6 or x > 6 => x > 6` [∵ x > 0]
APPEARS IN
RELATED QUESTIONS
The price of relatives and weights of a set of commodities are given below:
| Commodity | A | B | C | D |
| Price relatives | 125 | 120 | 127 | 119 |
| Weights | x | 2x | y | y + 3 |
If the sum of the weights is 40 and the weighted average of price relatives index number is 122, find the numerical values of x and y.
Construct 3 yearly moving averages from the following data and show on a graph against the original data:
| Year | 2000 | 2001 | 2002 | 2003 | 2004 | 2005 | 2006 | 2007 | 2008 | 2009 |
| Annual sales in lakhs | 18 | 22 | 20 | 26 | 30 | 22 | 24 | 28 | 32 | 35 |
The cost function C(x) = 3x2 – 6x + 5. Find the average cost when x = 2.
The Average Cost function associated with producing and marketing x units of an item is given by AC = `x + 5 + 36/x`.
- Find the Total cost function.
- Find the range of values of x for which Average Cost is increasing.
Which condition is true if Average Cost (AC) is constant at all levels of output?
The cost function of a commodity is `C(x) = 200 + 20x - 1/2x^2` (in rupees). Find the range where AC falls.
