Advertisements
Advertisements
Question
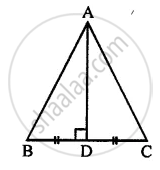
The given figure shows a triangle ABC in which AD is perpendicular to side BC and BD = CD. Prove that:
(i) ∆ ABD ≅ ∆ ACD
(ii) AB = AC
(iii) ∠B = ∠C
Solution
(i) In the given figure Δ ABC
AD ⊥ BC, BD = CD
In Δ ABD and Δ ACD
AD = AD ............(common)
∠ADB = ∠ADC ...............(each 90°)
BD = CD ...........(Given)
∴ Δ ABD ≅ Δ CAD .........(By SAS Rule)
(ii) Side AB = AC .........(c.p.c.t.)
(iii) ∠B = ∠C
Reason, since Δ ADB ≅ Δ ADC
∴ ∠B = ∠C
Hence proved.
APPEARS IN
RELATED QUESTIONS
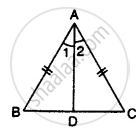
In the given figure, prove that: BD = BC.
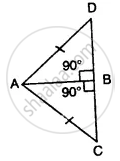
In the given figure ;
∠1 = ∠2 and AB = AC.
Prove that:
(i) ∠B = ∠C
(ii) BD = DC
(iii) AD is perpendicular to BC.
In the given figure prove that:
(i) PQ = RS
(ii) PS = QR
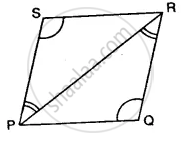
(i) ∆ XYZ ≅ ∆ XPZ
(ii) YZ = PZ
(iii) ∠YXZ = ∠PXZ

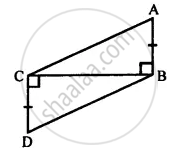
In the given figure, prove that:
(i) ∆ ABC ≅ ∆ DCB
(ii) AC = DB
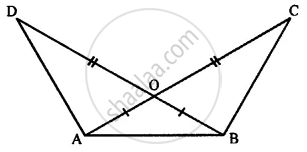
In the given figure, prove that:
(i) ∆ AOD ≅ ∆ BOC
(ii) AD = BC
(iii) ∠ADB = ∠ACB
(iv) ∆ ADB ≅ ∆ BCA
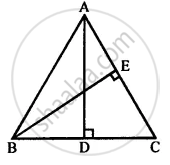
ABC is an equilateral triangle, AD and BE are perpendiculars to BC and AC respectively. Prove that:
(i) AD = BE
(ii) BD = CE
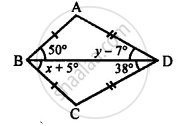
Use the information given in the following figure to prove triangles ABD and CBD are congruent. Also, find the values of x and y.