Question

The given figure shows a beam AB hinged at A and roller supported at B. The L shaped portion is welded at D to the beam AB. For the loading shown,find the support reactions.

Given : Beam AB hinged at A and roller supported at B and different forces acting on it.
To find : Support reactions

Answer 1

 

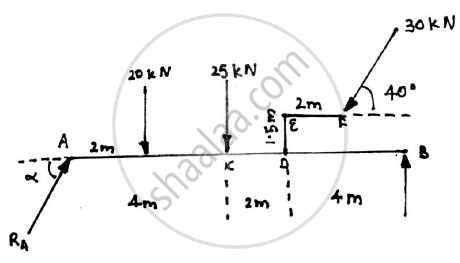
Force of distributed load AC = 5 x 4
                                             = 20 kN
Distance of force acting from point A =` 4/2` =2m
The beam is in equilibrium
Applying the conditions of equilibrium

ΣM_A = 0 
-20 x 2 – 25 x 4 - 30sin40 x 8 + 30cos40 x 1.5 + RB x 10 = 0
10R_B = 40 + 100 + 240sin40 - 45cos40 
10R_B = 259.797 kN 
R_B = 25.9797 kN (Acting upwards) 
Applying the conditions of equilibrium

ΣF_X = 0  
R_AX -30cos40 = 0 
R_AX = 22.9813 kN ………(1)

ΣF_Y = 0 

R_AY – 20 – 25 - 30sin40 + RB = 0

R_AY= 38.3039 kN ………..(2) 

`R_A = sqrt(R_(AX)^2 + R_(AY)^2 )`

`R_A = sqrt( 22.9813^2+38.3039^2)`

`R_A = 44.6691   kN`
`α =tan-1(R_(AY))/(R_(AX))`
`=tan-1(38.3039)/(22.9813)`
α=59.0374^o

Reaction at hinge A = 44.6691 kN (59.0374^o in first quadrant)
Reaction at roller B = 25.9797 kN (Towards up)