Advertisements
Advertisements
Question
The measure of each exterior angle of a regular pentagon is ______.
Solution
The measure of each exterior angle of a regular pentagon is 72°.
Explanation:
Measure of exterior angle
= `360^circ/"Number of sides"`
= `360^circ/5` ...[∵ In pentagon, number of sides, n = 5]
= 72°
APPEARS IN
RELATED QUESTIONS
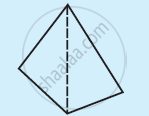
Examine the table. (Each figure is divided into triangles and the sum of the angles deduced from that.)
| Figure | 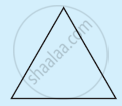 |
 |
 |
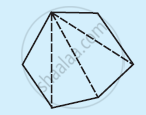 |
| Side | 3 | 4 | 5 | 6 |
| Angle sum | 180° |
2 × 180° = (4 − 2) × 180° |
3 × 180° = (5 − 2) × 180° |
4 × 180° = (6 − 2) × 180° |
What can you say about the angle sum of a convex polygon with number of sides?
a) 7
b) 8
c) 10
d) n
Is it possible to have a regular polygon with measure of each exterior angle as 22°?
Classify the following curve as open or closed:

Find the number of side of a regular polygon, when of its angle has a measure of 162° .
Find the number of degrees in each exterior exterior angle of a regular pentagon.
How many diagonals does a hexagon have?
The number of sides of a regular polygon whose each interior angle is of 135° is ______.
The measure of each angle of a regular pentagon is ______.
A regular polygon is a polygon whose all sides are equal and all ______ are equal.
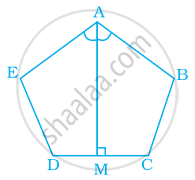
ABCDE is a regular pentagon. The bisector of angle A meets the side CD at M. Find ∠AMC.