Advertisements
Advertisements
Question
The perimeter of a rectangle is 240 cm. If its length is increased by 10% and its breadth is decreased by 20%, we get the same perimeter. Find the length and breadth of the rectangle.
Solution
Given, perimeter of rectangle = 240 cm
⇒ 2 × (Length + Breadth) = 240 cm
⇒ Length + Breadth = 120 cm
Let the length of rectangle be x cm
Then, the breadth of rectangle = (120 – x) cm
∴ New length of rectangle = x + 10% of x
= `x + 10/100 xx x`
= `110/100 x cm`
And new breadth of rectangle = (120 – x) – 20% of (120 – x) cm
= `(120 - x) - 20/100 xx (120 - x)`
= `(120 - x)(1 - 20/100)`
= `80/100 (120 - x) cm`
It is given that, perimeter of new rectangle is 240 cm.
According to the question,
2 × (New length + New breadth) = 240
⇒ `2 xx [(110x)/100 + 80/100 (120 - x)] = 240`
⇒ `(110x + 9600 - 80x)/100 = 240/2`
⇒ `30x + 9600 = 240/2 xx 100`
⇒ 30x + 9600 = 12000
⇒ 30x = 12000 – 9600
⇒ 30x = 2400
⇒ x = `2400/30` = 80
Hence, length of rectangle = 80 cm
And breadth of rectangle = 120 – 80 = 40 cm.
APPEARS IN
RELATED QUESTIONS
The number of boys and girls in a class are in the ratio 7:5. The number of boys is 8 more than the number of girls. What is the total class strength?
A rational number is such that when you multiply it by `5/2`and add `2/3` to the product, you get `-7/12`. What is the number?
The perimeter of a rectangle is 13 cm and its width is `2 3/4` cm. Find its length.
Bansi has 3 times as many two-rupee coins as he has five-rupee coins. If he has in all a sum of Rs. 77, how many coins of each denomination does he have?
3 years ago, the age of a boy was y years. His age 2 years ago was (y – 2) years.
The sum of three consecutive even natural numbers is 48. Find the greatest of these numbers.
An employee works in a company on a contract of 30 days on the condition that he will receive Rs. 120 for each day he works and he will be fined Rs. 10 for each day he is absent. If he receives Rs. 2300 in all, for how many days did he remain absent?
For what value of x is the perimeter of shape 77 cm?
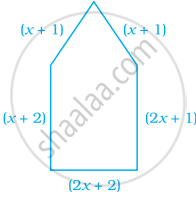
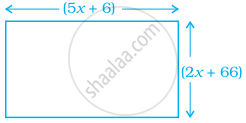
For what value of x is the perimeter of shape 186 cm?
On dividing Rs. 200 between A and B such that twice of A’s share is less than 3 times B’s share by 200, B’s share is?
