Advertisements
Advertisements
Question
The perimeter of a triangle is 14.4 cm and the ratio of lengths of its side is 2 : 3 : 4. Construct the triangle.
Advertisements
Solution 1
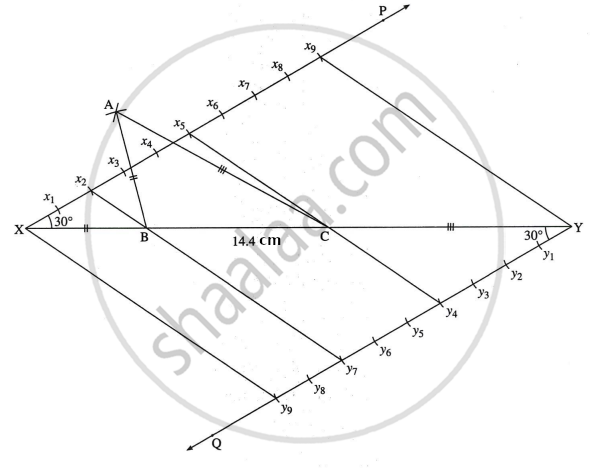
Explanation:
Let ΔABC be the required triangle.
Draw the line XY = 14.4 cm, which is the perimeter of ΔABC.
Divide line XY in the ratio 2:3:4.
Draw ray XP such that, ∠YXP = 30°.
Draw line YQ on the opposite side of ray XP such that, `angle`XYQ = 30°.
Make ray XP into 9 equal parts i.e. `"X"_(x_1)`,x1x2, ... x8x9.
Similarly, according to the figure, make 9 equal parts of ray YQ.
Join x2y7 and x5y4, intersecting line XY at B and C respectively.
Draw an arc considering B as center and BX as radius.
Taking C as center and CY as radius, draw an arc which intersects the first arc at point A.
Draw line AB and line AC.
∴ ΔABC is the required triangle.
Solution 2
Rough figure:
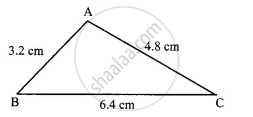
Explanation:
Let the common multiple be x
∴ In ∆ABC,
AB = 2x cm, AC = 3x cm, BC = 4x cm
Perimeter of triangle = 14.4 cm
∴ AB + BC + AC = 14.4
∴ 9x = 14.4
∴ `x = 14.4/9`
∴ x = 1.6
∴ AB = 2x = 2 × 1.6 = 3.2 cm
∴ AC = 3x = 3 × 1.6 = 4.8 cm
∴ BC = 4x = 4 × 1.6 = 6.4 cm
APPEARS IN
RELATED QUESTIONS
In ∆ STU, l(ST) = 7 cm, l(TU) = 4 cm, l(SU) = 5 cm
Draw an isosceles triangle with base 5 cm and the other sides 3.5 cm each.
Draw an equilateral triangle with side 6.5 cm.
Construct a triangle of the measures given below.
In ∆SAT, l(AT) = 6.4 cm, m ∠A = 45°, m ∠T = 105°
Construct a triangle of the measures given below.
Students should take examples of their own and practise the construction of triangles.
Construct ∆ABC such that m∠A = 55°, m∠B = 60°, and l(AB) = 5.9 cm.
Construct a triangle using the given data: BC = 6cm, AC = 5.0cm and ∠C = 60°
Construct a triangle using the given data: XY = 5.2cm, XZ = 6.5cm and ∠X = 75°
Construct a triangle using the given data: PQ = 6.2cm, QR = 9.0cm and ∠Q = 30°
Construct a right-angled triangle in which: QP = QR and hypotenuse PR = 7 cm
Construct an isosceles triangle in which: PQ = QR, PR = 4.5 cm and ∠R = 60°
Construct a triangle using the following data: XY + YZ = 5.6 cm, XZ = 4.5 cm and ∠X = 45°
Construct a triangle using the given data: AB - AC = 1.2 cm, BC = 6.0 cm and ∠B = 60°
Construct a triangle using the given data: Perimeter of triangle is 10.6 cm, and the base angles are 60° and 45°
Construct a ΔXYZ with YZ = 7.5 cm, ∠Y = 60° and ∠Z = 45°. Draw the bisectors of ∠Y and ∠Z. If these bisectors meet at O, measure angle YOZ.
Construct a triangle PQR with PQ = 5.4 cm, QR = 4.6 cm and ∠Q = 60°. Draw the perpendicular PS at QR, measure the lengths of SP and SQ,
In Construction of triangle ABC where AB = 3 cm, BC = 5 cm, and ∠ABC = 60°, which side must be drawn first as the base?
For Construction of △ABC where AB = 4 cm, ∠A = 60°, and ∠B = 30°, what is the critical step used to locate vertex C?
