Advertisements
Advertisements
Question
Two bodies of masses m and 4m are placed at a distance of r. Calculate the gravitational potential at a point on the line joining them where the gravitational field is zero.
Solution
Let the point be the position when the gravitational field is zero.,
`("Gm")/"x"^2 = ("G"(4"m"))/("r" - "x")^2`
`1/"x"^2 = 4/("r" - "x")^2`
Square root on both sides,
`1/"x" = 2/(("r" - "x"))`
∴ x = `"r"/3`
The point P is at a distance `"r"/3` from mass ‘m’ and `"2r"/3` from mass ‘4m’
Gravitational potential V = `(-"Gm")/(("r"/3)) - ("G"(4"m"))/(("2r"/3))`
= `(-3"Gm")/4 - (3"G"(4"m"))/(2"r")`
= `(-3"Gm")/"r" - (12"Gm")/(2"r")`
V = `(-9"Gm")/"r"`
APPEARS IN
RELATED QUESTIONS
If the distance between the Earth and Sun were to be doubled from its present value, the number of days in a year would be ___________.
The gravitational potential energy of the Moon with respect to Earth is ____________.
If the mass and radius of the Earth are both doubled, then the acceleration due to gravity g'
Write unit of the gravitational field.
Define gravitational potential energy.
What is the difference between gravitational potential and gravitational potential energy?
If a comet suddenly hits the Moon and imparts energy which is more than the total energy of the Moon, what will happen?
If the Earth’s pull on the Moon suddenly disappears, what will happen to the Moon?
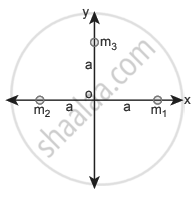
Calculate the gravitational field at point O due to three masses m1, m2 and m3 whose positions are given by the following figure. If the masses m1 and m2 are equal what is the change in a gravitational field at the point O?
Earth revolves around the Sun at 30 km s−1. Calculate the kinetic energy of the Earth. In the previous example, you calculated the potential energy of the Earth. What is the total energy of the Earth in that case? Is the total energy positive? Give reasons.
