Advertisements
Advertisements
Question
Two resistors P and Q of the same material and length but of different thickness are connected in parallel to a battery. The cross- sectional area of P is twice that of Q. What is the ratio of
(a) The resistance of P to the resistance of Q?
(b) The current in P to the current in Q?
Solution
We know that, R = ρ `1/"a"`
(a) Given length of resistors P and Q made of same material is same (say= 1)
Let 'a' be the area of cross-section of Q.
Then, the area of cross-section of Pis '2a'.
`"R"_"P"/"R"_"Q" = [ρ (1/[2"a"])]/[ρ (1/"a")] = 1/2`
the required ratio is 1: 2.
(b) AJC to ohm's law,
V=IR
If resistors P and Q are connected across the same voltage supply V, then
`"I"_"P"/"I"_"Q" = "R"_"g"/"R"_"p" = 2/1`
:. the required ratio of current in P to current in Qi s 2: 1.
APPEARS IN
RELATED QUESTIONS
What does an electric circuit mean?
Draw the electrical symbols of the following components and state its use:
Ammeter.
The atoms of copper contain electrons and the atoms of rubber also contain electrons. Then why does copper conduct electricity but rubber does not conduct electricity?
A car headlight bulb working on a 12 V car battery draws a current of 0.5 A. The resistance of the light bulb is:
(a) 0.5 Ω
(b) 6 Ω
(c) 12 Ω
(d) 24 Ω
The diagram shows two ways of connecting three lamps P, Q and R to A.C. supply of 220 V.
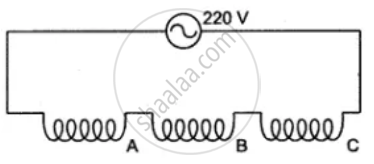
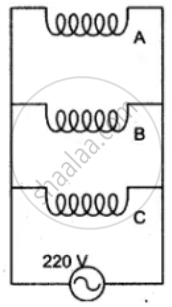
1 kWh = `(1 "volt" xx 1 "ampere" xx "_________")/1000`
A current of 0.2 A flows through a wire whose ends are at a potential difference of 15 V. Calculate:
(i) The resistance of the wire, and
(ii) The heat energy produced in 1 minute.
