Advertisements
Advertisements
Question
Use (i) the Ampere’s law for H and (ii) continuity of lines of B, to conclude that inside a bar magnet, (a) lines of H run from the N pole to S pole, while (b) lines of B must run from the S pole to N pole.
Solution
Let us consider a magnetic field line of B through the bar magnet as given in the figure below. It must be a closed loop.
Consider an Amperian loop C inside and outside the amgnet NS on side PQ of magnet then `int_P^Q vecH .vec(dl) = int_Q^P vecB/mu_0 vec(dl)`
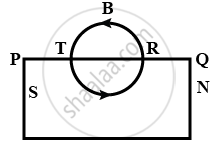
Where B is magnetic field and m0 is dipole moment. As angle between B and dl varies from 90°, 0, 90° from R to T in figure, so cos θ is greater than 1. So `int_P^Q vecH. vec(dl) = int_Q^P vecB/mu_0 .vec(dl) > 0` i.e. posotive.
Hence, the value of B must be varied from south pole to north pole inside the magnet.
According to Ampere's law `oint_(PQP) vecH.vec(dl) = 0`
`oint_(pQP) vecH.vec(dl) = int_P^Q vecH.vec(dl) + int_Q^P vecH.vec(dl) = 0`
As `int_P^Q H.dl > 0` (outside the magnet) and `int_Q^P H.dl > 0` (inside the magnet). It is due to the angle between H and dl is more than 90° inside the magnet so cos θ is negative. It means the lines of H must run from north pole to south pole.
APPEARS IN
RELATED QUESTIONS
How are the magnetic field lines different from the electrostatic field lines?
Predict the polarity of the capacitor in the situation described below :

A bar magnet of length 1 cm and cross-sectional area 1.0 cm2 produces a magnetic field of 1.5 × 10−4 T at a point in end-on position at a distance 15 cm away from the centre. (a) Find the magnetic moment M of the magnet. (b) Find the magnetisation I of the magnet. (c) Find the magnetic field B at the centre of the magnet.
Choose the correct option.
Inside a bar magnet, the magnetic field lines
Solve the following problem.
Two small and similar bar magnets have a magnetic dipole moment of 1.0 Am2 each. They are kept in a plane in such a way that their axes are perpendicular to each other. A line drawn through the axis of one magnet passes through the center of other magnet. If the distance between their centers is 2 m, find the magnitude of the magnetic field at the midpoint of the line joining their centers.
Magnetic moment for solenoid and corresponding bar magnet is ______.
A bar magnet of magnetic moment 3.0 Am is placed in a uniform magnetic field of 2 × 10-5T. If each pole of the magnet experience a force of 6 × 10-4 N, the length of the magnet is ______.
A toroid of n turns, mean radius R and cross-sectional radius a carries current I. It is placed on a horizontal table taken as x-y plane. Its magnetic moment m ______.
There are two current carrying planar coils made each from identical wires of length L. C1 is circular (radius R) and C2 is square (side a). They are so constructed that they have same frequency of oscillation when they are placed in the same uniform B and carry the same current. Find a in terms of R.
A long straight wire of circular cross section of radius 'a' carries a steady current I. The current is uniformly distributed across its cross section. The ratio of magnitudes of the magnetic field at a point `a/2` above the surface of wire to that of a point `a/2` below its surface is ______.
