Question

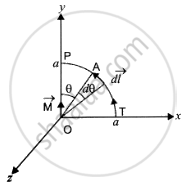
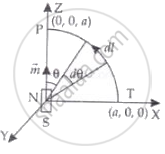
Verify the Ampere’s law for magnetic field of a point dipole of dipole moment m = m`hatk`. Take C as the closed curve running clockwise along (i) the z-axis from z = a > 0 to z = R; (ii) along the quarter circle of radius R and centre at the origin, in the first quadrant of x-z plane; (iii) along the x-axis from x = R to x = a, and (iv) along the quarter circle of radius a and centre at the origin in the first quadrant of x-z plane.

Answer 1

Consider a plane on x-z plane on which there are two loops (of radius R and a)and a point dipole on origin of dipole moment M(as shown in the figure). From P to Q, every point on the z-axis lies at the axial line of magnetic dipole of moment M.

So magnetic field induction (B) at a point (0, 0, z) from magnetic dipole (centre at origin) and having magnetic moment `vecm hatk` of magnitude `(vecm)`.

`|B| = mu_0/(4pi) (2(vecm))/z^3 = (mu_0vecm)/(2piz^3)`

(i) Ampere's law along Z axis from Z = a to Z = R i.e. from P to Q

`int_P^Q vecB.vec(dl) = int_P^Q B.dl  cos θ^circ = int_P^Q B.dz`

= `int_P^Q (mu_0vecm)/(2piz^3) dz = (mu_0vecm)/(2pi) int_a^R z^(-3) dz`  .....[∵ Distance of P and Q from origin are a and R respectively]

= `(mu_0vecm)/(2pi) [z^-2/(-2)]_a^R = (mu_0vecm)/(2pi(-2)) [1/R^2 - 1/a^2]`

`int_P^Q B.dl = (mu_0vecm)/(4pi) [1/a^2 - 1/R^2]`

(ii) Ampere's law along the quarter circle QS of radius R as given in figure here. Point A can be considered on the equatorial line of magnetic dipole of moment `vecm` sin θ.

Magnetic field of point A on the circular are is

`B = mu_0/(4pi) (vecm  sin theta)/R^3`

`d theta = (dl)/R` ⇒ `R.d  theta`

∴ By Ampere's law `int B.dl = int B.dl cos theta`

`int vecB. vec(dl) = int_0^(pi/2) mu_0/(4pi) (vecm  sin theta)/R^3 * R.d  theta`

`int vecB.vec(dl) = (mu_0vecm)/(4piR^2) [- cos  theta]_0^(pi/2) = (mu_0vecm)/(4piR^2) [- cos 90^circ + cos theta^circ]`

`int B.dl = (mu_0vecm)/(4piR^2)`

(iii) Ampere's law along the X-axis from x = R to x = a as in given figure here.

As all point from S to T lies on equatorial line of magnetic dipole N-S. So magnetic field induction at a point P at a distance x from the dipole is

`B = mu_0/(4pi x^3) = mu_0/(4pi) (vecm hatk)/x^3`

`int vecB.vec(dl) = int_R^q (- mu_0 vecmhatk)/(4 pix^3) * vec(dl)`  

∵ Angle between dl and m is 90°

So `int vecB.vec(dl) = int_R^a (- mu_0 |vecm| dl  cos 90^circ)/(4pix^3)` = 0

`B = mu_0/(4pi) vecm/x^3 = mu_0/(4pi) (vecm hatk)/x^3`

`int vecB.vec(dl) = int_R^a (- mu_0 vecmhatk)/(4pix^3) * vec(dl)`

∵ Angle between dl and m is 90°

So `int vecB.vec(dl) = int_R^a (- mu_0 |vecm| dl  cos 90^circ)/(4pix^3)` = 0

(iv) Ampere's law along the quarter circle of radius 'a' and centre at the origin in the quadrant X-z plane as in figure here as on part (ii).

`int B.dl = int_(pi/2)^0 mu_0/(4pi) (vecm  sin theta)/a^3 * ad  theta`

`int B.dl = (mu_0vecm)/(4pia^2) [- cos  theta]_(pi/2)^0`

θ = angle from axial line of dipole

`int B.dl = (mu_0vecm)/(4pia) [- cos theta + cos  pi/2] = (mu_0vecm)/(4pia^2) (-1 + 0) = (-mu_0vecm)/(4pia^2)`

∴ Applying Ampere's law along close path starting from P to Q, Q to S and S to P.

`oint_(PQST) B.dl = int_P^Q vecB. vec(dl) + int_Q^S vecB.vec(dl) + int_S^T vecB. vec(dl) + int_T^P B.dl`

From parts (i), (ii), (iii) and (iv) substituting the values

`oint_(PQST) B.dl = (mu_0vecm)/(4pi) (1/a^2 - 1/R^2) + (mu_0vecm)/(4piR^2) + 0 + (-mu_0vecm)/(4pia^2)`

= `(mu_0vecm)/(4pia^2) - (mu_0vecm)/(4piR^2) + (mu_0vecm)/(4piR) - (mu_0vecm)/(4pia^2)`

`oint_(PQST) B.dl` = 0

This proves that Ampere's law is magnetism.