Advertisements
Advertisements
Question
Volume of a cuboid is 12 cm3. The volume (in cm3) of a cuboid whose sides are double of the above cuboid is
Options
24
48
72
96
Solution
Let,
l → Length of the first cuboid
b → Breadth of the first cuboid
h → Height of the first cuboid
Volume of the cuboid is 12 cm3
Dimensions of the new cuboid are,
Length (L) = 2l
Breadth (B) = 2b
Height (H) = 2h
We are asked to find the volume of the new cuboid
We know that,
Volume of the new cuboid,
V' = LBH
= (2l)(2b)(2h)
= 8(lbh)
= 8V { Sincr , V = lbh}
= 8 × 12 { Since , V = 12 cm 3 }
= 96 cm3
Thus volume of the new cuboid is 96 cm3.
APPEARS IN
RELATED QUESTIONS
The paint in a certain container is sufficient to paint an area equal to 9.375 m2. How many bricks of dimensions 22.5 cm × 10 cm × 7.5 cm can be painted out of this container?
An open box is made of wood 3 cm thick. Its external length, breadth and height are 1.48 m, 1.16 m and 8.3 m. Find the cost of painting the inner surface of Rs 50 per sq. metre.
The weight of a metal block of size 5 cm by 4 cm by 3 cm is 1 kg. Find the weight of a block of the same metal of size 15 cm by 8 cm by 3 cm.
If V is the volume of a cuboid of dimensions a, b, c and S is its surface area, then prove that \[\frac{1}{V} = \frac{2}{S}\left( \frac{1}{a} + \frac{1}{b} + \frac{1}{c} \right)\]
The volume of a cube whose surface area is 96 cm2, is
If each edge of a cube, of volume V, is doubled, then the volume of the new cube is
The length, breadth, and height of a rectangular solid are in the ratio 6 : 4 :3. If the total surface area is 1728 cm2. Find its dimensions.
The dimensions of a cuboidal box are 6 m × 400 cm × 1.5 m. Find the cost of painting its entire outer surface at the rate of ₹ 22 per m2.
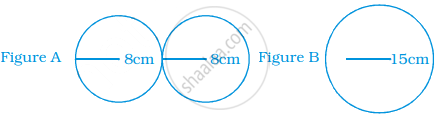
Below are the drawings of cross sections of two different pipes used to fill swimming pools. Figure A is a combination of 2 pipes each having a radius of 8 cm. Figure B is a pipe having a radius of 15 cm. If the force of the flow of water coming out of the pipes is the same in both the cases, which will fill the swimming pool faster?