Advertisements
Advertisements
Question
With a neat labelled diagram and derive the equation for three resistances connected in parallel.
Solution
When two or more resistors are joined at the same end, the resistances are connected in parallel.

The potential difference in parallel remains the same across all the resistors.
The current is the sum of the currents across all the individual resistors.
l = l1 + l2 + l3 .........(1)
Let Rp be the resultant resistance of the circuit.
On applying Ohm’s law to the entire circuit, we get
l = V/Rp ................(2)
Now, applying Ohm’s law to individual resistances, we get
l1 = V/R1
l2 = V/R2
l3 = V/R3 ................(3)
From equations (1), (2) and (3), we get
`V/R_p=V/R_1+V/R_2+V/R_3`
`therefore1/R_p=1/R_1+1/R_2+1/R_3`
Here, Rp is the resultant resistance. Thus, the reciprocal of the resultant resistance of a parallel combination of resistors is the sum of the reciprocals of individual resistances. The resultant resistance is lesser than all the resistances.
APPEARS IN
RELATED QUESTIONS
An electric lamp of 100 Ω, a toaster of resistance 50 Ω, and a water filter of resistance 500 Ω are connected in parallel to a 220 V source. What is the resistance of an electric iron connected to the same source that takes as much current as all three appliances, and what is the current through it?
Show how you would connect three resistors, each of resistance 6 Ω, so that the combination has a resistance of 9 Ω.
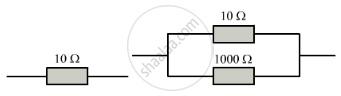
Which of the following arrangement, A or B, has the lower combined resistance?
Two resistors, with resistance 5 Ω and 10 Ω respectively are to be connected to a battery of emf 6 V so as to obtain:
(i) minimum current flowing
(ii) maximum current flowing
(a) How will you connect the resistances in each case?
(b) Calculate the strength of the total current in the circuit in the two cases.
Explain with the help of a labelled circuit diagram, how you will find the resistance of a combination of three resistors of resistances R1, R2 and R3 joined in parallel.
How will you connect three resistors of resistances 2 Ω, 3 Ω, and 6 Ω to obtain a total resistance of 4 Ω, and 1 Ω?
State how are the two resistors joined with a battery when potential difference is same across each resistor.
Four resistors each of resistance 2Ω are connected in parallel. What is the effective resistance?
A particular resistance wire has a resistance of 3 ohm per meter. Find the total resistance of three lengths of this wire each 1.5 m long, joined in parallel.
A battery of e.m.f. 15 V and internal resistance 2 `Omega` is connected to tvvo resistors of 4 ohm and 6 ohm joined.
(i) In series,
(ii) In para 1 lel. Find in each case the electrica I energy spent per minute in 6 ohm resistor.
The equivalent resistance of a parallel combination of two resistors of 30 Ω and 60 Ω is ______________.
How does the resistance of a metallic wire depend on the length of wire?
Calculate equivalent resistance in the following cases:
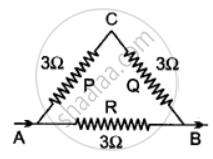
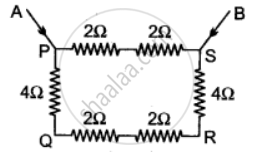
Two devices are connected between two points say A and B in parallel. The physical quantity that will remain the same between the two points is:
The least resistance obtained by using 2 Ω, 4 Ω, 1 Ω and 100 Ω is:
Three resistors of 1 Ω, 2 Ω and 3 Ω are connected in parallel. The combined resistance of the three resistors should be:
You are given four ammeters A, B, C and D having the least counts mentioned below:
(I) Ammeter A with least count 0.25 A
(II) Ammeter B with least count 0.5 A
(III) Ammeter C with least count 0.05 A
(IV) Ammeter D with least count 0.1 A
Which of the ammeters would you prefer for doing an experiment to determine the equivalent resistance of two resistances most accurately, when connected in parallel?

The diagram above is a schematic diagram of a household circuit. The house shown in the above diagram has 5 usable spaces where electrical connections are made. For this house, the mains have a voltage of 220 V and the net current coming from the mains is 22A.
- What is the mode of connection to all the spaces in the house from the mains?
- The spaces 5 and 4 have the same resistance and spaces 3 and 2 have respective resistances of 20Ω and 30Ω. Space 1 has a resistance double that of space 5. What is the net resistance for space 5.
- What is the current in space 3?
- What should be placed between the main connection and the rest of the house’s electrical appliances to save them from accidental high electric current?
Two resistors of resistance 2 Ω and 3 Ω are connected in parallel to a cell to draw current 0.5 A from the cell. Calculate the current in each resistor.
