Advertisements
Advertisements
Question
Write down the equation of the time period for the linear harmonic oscillator.
Solution
From Newton’s second law, we can write the equation for the particle executing simple harmonic motion
`"m"("d"^2"x")/("dt"^2)` = −kx ............(1)
`("d"^2"x")/("dt"^2) = -"k"/"m""x"` .............(2)
Comparing the equation with simple harmonic motion equation, we get
`ω^2 = "k"/"m"` .............(3)
which means the angular frequency or natural frequency of the oscillator is
`ω = sqrt("k"/"m")` rad s−1 ............(4)
The frequency of the oscillation is
f = `ω/(2π) = 1/(2π) sqrt("k"/"m")` Hertz ...........(5)
and the time period of the oscillation is
T = `1/"f" = 2π sqrt("m"/"k")` seconds ........(6)
APPEARS IN
RELATED QUESTIONS
Two bodies A and B whose masses are in the ratio 1 : 2 are suspended from two separate massless springs of force constants kA and kB respectively. If the two bodies oscillate vertically such that their maximum velocities are in the ratio 1 : 2, the ratio of the amplitude A to that of B is
A spring is connected to a mass m suspended from it and its time period for vertical oscillation is T. The spring is now cut into two equal halves and the same mass is suspended from one of the halves. The period of vertical oscillation is
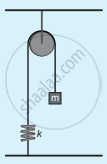
The time period for small vertical oscillations of block of mass m when the masses of the pulleys are negligible and spring constant k1 and k2 is

An ideal spring of spring constant k, is suspended from the ceiling of a room and a block of mass M is fastened to its lower end. If the block is released when the spring is un-stretched, then the maximum extension in the spring is
Discuss the simple pendulum in detail.
Explain the horizontal oscillations of a spring.
Describe the vertical oscillations of a spring.
Write short notes on the oscillations of the liquid column in the U-tube.
A piece of wood of mass m is floating erect in a liquid whose density is ρ. If it is slightly pressed down and released, then executes simple harmonic motion. Show that its time period of oscillation is T = `2π sqrt("m"/("Ag"ρ))`
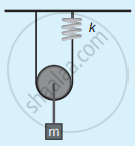
Compute the time period for the following system if the block of mass m is slightly displaced vertically down from its equilibrium position and then released. Assume that the pulley is light and smooth, strings and springs are light.