Resolution of Vectors

We should know that there are two rectangular components for a vector, i.e. the horizontal component and the vertical component, the horizontal component lies on the x-axis whereas the vertical component lies on the y-axis,

Think of it this way; the horizontal component will resemble the shadow of the vector r falling on the x-axis if the light were shining from above. Similarly, the vertical component will resemble the shadow of vector r falling on the y-axis if the light were shining from the side.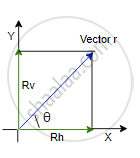

Now let us call the vertical component `bar r_v` and the horizontal vector as `bar r_h` and let us call the angle made by the vector `bar r` with the horizontal component as θ.
If we notice carefully the 3 vector `bar r_v bar r_h and bar r`form the 3 sides of a right angled traingle, so from the trigonometry we can say that,
`|bar r_h| = | bar r| cos θ `
The reason is for the angle θ r is the hypotenuse and `r_h`is the adjacent side, so adj/hyp = cosine of the angle, so from this rule we can find the magnitude of the horizontal vector given that we know the magnitude of the vector r and the angle it makes with the horizontal vector.

Similarly, the magnitude of the vertical component can be found using the sine function because vertical component resembles the opposite side of the triangle and opp/hyp = sine of the angle, thereby the magnitude of the vertical component is given by,
`|bar r_v| = | bar r| sin θ`
Now that we know how to get the magnitude of the rectangular components of the two vectors how do we find out the direction and the magnitude of the resultant vector if its horizontal and vertical components are given, this could be done easily with a graphical method,

Imagine we have the horizontal component of magnitude 100 Newtons and a vertical component of magnitude 40 Newtons then we can draw a right-angled triangle with the given data. By plotting the lengths of the vectors proportional to their magnitude. i.e.

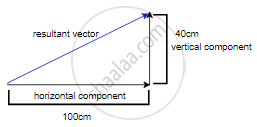
Now the resultant vector could be drawn as the hypotenuse, and the length of the vector gives us the magnitude of the resultant vector as well as its direction.

Consider the following vector r; the vector r can be resolved into horizontal and vertical components, these two components add up to give us the resultant vector i.e. vector r as shown in the figure above.

A unit vector is a vector of unit magnitude and points in a particular direction. It is used to specify the direction only. Unit vector is represented by putting a cap (^) over the quantity.
The unit vector in the direction of `barA` is denoted by `Â` and defined by
`Â= (barA)/(|barA|)=(barA)/A or barA=AÂ`