Advertisements
Advertisements
प्रश्न
A block of mass m sliding on a smooth horizontal surface with a velocity \[\vec{\nu}\] meets a long horizontal spring fixed at one end and with spring constant k, as shown in following figure following. Find the maximum compression of the spring. Will the velocity of the block be the same as \[\vec{\nu}\] when it comes back to the original position shown?

उत्तर
Let the compression in the spring be x.
(a) Applying the law of conservation of energy,
maximum compression in the spring will be produced when the block comes to rest .
so change in kinetic energy of the block due to change in its velocity from u m/s to 0 will be equal to the gain in potential energy of the spring.
change in kinetic energy of the block= \[\frac{1}{2}\text{mv}^2 - \frac{1}{2}\text{m}(0 )^2 = \frac{1}{2}\text{mv}^2\]
gain in the potential energy of spring= \[\frac{1}{2}\text{kx}^2\]
\[\frac{1}{2}\text{m} \nu^2 = \frac{1}{2}\text{kx}^2 \]
\[ \Rightarrow \text{x}^2 = \frac{\text{m} \nu^2}{\text{k}}\]
\[\text{x} = \nu\sqrt{\left( \frac{\text{m}}{\text{k}} \right)}\]
(b) No. The velocity of the block will not be same when it comes back to the original position. It will be in the opposite direction and the magnitude will be the same if we neglect all losses due friction and spring to be perfectly elastic.
APPEARS IN
संबंधित प्रश्न
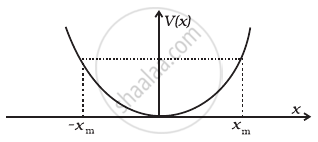
The potential energy function for a particle executing linear simple harmonic motion is given by V(x) =`(kx^2)/2`, where k is the force constant of the oscillator. For k = 0.5 N m–1, the graph of V(x) versus x is shown in the figure. Show that a particle of total energy 1 J moving under this potential must ‘turn back’ when it reaches x = ± 2 m.

When a conservative force does positive work on a body, the potential energy of the body ______.
Two equal masses are attached to the two ends of a spring of spring constant k. The masses are pulled out symmetrically to stretch the spring by a length x over its natural length. The work done by the spring on each mass is
One end of a light spring of spring constant k is fixed to a wall and the other end is tied to a block placed on a smooth horizontal surface. In a displacement, the work done by the spring is \[\frac{1}{2}k x^2\] . The possible cases are
(a) at spring was initially compressed by a distance x and was finally in its natural length
(b) it was initially stretched by a distance x and and finally was in its natural length
(c) it was initially in its natural length and finally in a compressed position
(d) it was initially in its natural length and finally in a stretched position.
Following figure shows a spring fixed at the bottom end of an incline of inclination 37°. A small block of mass 2 kg starts slipping down the incline from a point 4⋅8 m away from the spring. The block compresses the spring by 20 cm, stops momentarily and then rebounds through a distance of 1 m up the incline. Find (a) the friction coefficient between the plane and the block and (b) the spring constant of the spring. Take g = 10 m/s2.

A block of mass m moving at a speed ν compresses a spring through a distance x before its speed is halved. Find the spring constant of the spring.
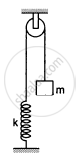
Consider the situation shown in the following figure. Initially the spring is unstretched when the system is released from rest. Assuming no friction in the pulley, find the maximum elongation of the spring.
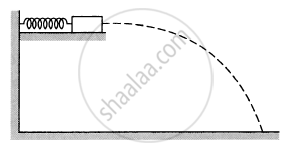
A small block of mass 100 g is pressed against a horizontal spring fixed at one end to compress the spring through 5 cm (figure following). The spring constant is 100 N/m. When released, the block moves horizontally till it leaves the spring. Where will it hit the ground 2 m below the spring?
If a spring extends by x on loading, then energy stored by the spring is (T is the tension in the spring and k is the spring constant)
The potential energy function for a particle executing linear SHM is given by `V(x) = 1/2 kx^2` where k is the force constant of the oscillator (Figure). For k = 0.5 N/m, the graph of V(x) versus x is shown in the figure. A particle of total energy E turns back when it reaches `x = ±x_m`. If V and K indicate the P.E. and K.E., respectively of the particle at `x = +x_m`, then which of the following is correct?
A body is moved along a closed loop. Is the work done in moving the body necessarily zero? If not, state the condition under which work done over a closed path is always zero.
Two identical steel cubes (masses 50 g, side 1 cm) collide head-on face to face with a speed of 10 cm/s each. Find the maximum compression of each. Young’s modulus for steel = Y = 2 × 1011 N/m2.
