Advertisements
Advertisements
प्रश्न
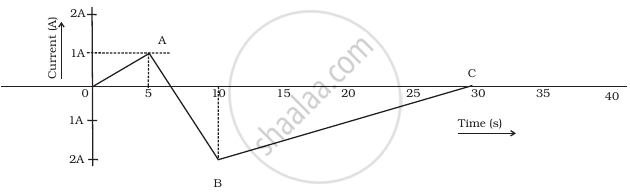
A (current vs time) graph of the current passing through a solenoid is shown in figure. For which time is the back electromotive force (u) a maximum. If the back emf at t = 3s is e, find the back emf at t = 7s, 15s and 40s. OA, AB and BC are straight line segments.
उत्तर
Whenever the electric current passing through a coil or circuit changes, the magnetic flux linked with it will also change. As a result of this, in accordance with Farraday's laws of electromagnetic induction, an emf is induced in the coil or the circuit which opposes the change that causes this induced emf is called back emf, the current so produced in the coil is called induced current. The induced emf is given by
`ε = - (d(Nphi_B))/(dt)`
`ε = - L (dl)/(dt)`
Thus, negative sign indicates that induced emf (e) opposes any change (increase or decrease) of current in the coil.
When the rate of change of current is maximum, then back emf in the solenoid is (u) a maximum. This occurs in AB part of the graph. So maximum back emf will be obtained between 5s < t < 10s.
Since, the back emf at t = 3s is e.
Also, the rate of change of current at t = 3
And slope(s) of OA (from t = 0s to t = 5s) = 1/5 A/s
So, we have
If u = L 1/5 (for t = 3s, `(dI)/(dt)` = 1/5)
Where L is a constant (coefficient of self-induction)
And emf is ε = `- L (dI)/(dt)`
Similarly, we have for other values
For 5s < t < 10s, u1 = `- L 3/5 = - 3/5 L = - 3e`
Thus, at t = 7s, u1 = – 3e
For 10s < t < 30s
u2 = `L 2/20 = L/10 = 1/2 e`
For t > 30s, u2 = 0
Thus, the back emf at t = 7s, 15s and 40s are – 3e e/2 and 0 respectively.
APPEARS IN
संबंधित प्रश्न
A jet plane is travelling towards west at a speed of 1800 km/h. What is the voltage difference developed between the ends of the wing having a span of 25 m, if the Earth’s magnetic field at the location has a magnitude of 5 × 10−4 T and the dip angle is 30°.
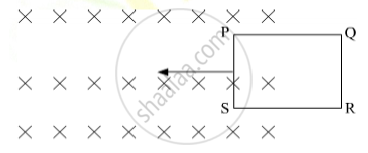
The closed loop (PQRS) of wire is moved into a uniform magnetic field at right angles to the plane of the paper as shown in the figure, Predict the direction of the induced current in the loop.
E°cell for the given redox reaction is 2.71V
\[\ce{Mg_{(s)} +Cu^{2+}_{(0.01 M)}->Mg^{2+}_{(0.001M)}+Cu_{(s)}}\]
Calculate Ecell for the reaction. Write the direction of flow of current when an external opposite potential applied is
(i) less than 2.71 V and
(ii) greater than 2.71 V
Faraday’s laws are consequence of the conservation of ______.
The average e.m.f induced in a coil in which current change from 0.2 ampere to 0.4 ampere in· 0.1 sec is 1 volt, the self-inductance of the coil is
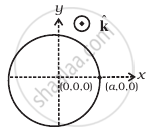
A magnetic field in a certain region is given by `B = B_o cos (ωt)hatk` and a coil of radius a with resistance R is placed in the x-y plane with its centre at the origin in the magnetic field (Figure) . Find the magnitude and the direction of the current at (a, 0, 0) at t = π/2ω, t = π/ω and t = 3π/2ω.
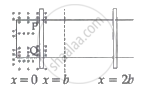
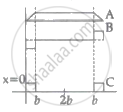
The arm PQ of a rectangular conductor is moving from x = 0 to x = 2b outwards and then inwards from x = 2b to x = 0 as shown in the figure. A uniform magnetic field perpendicular to the plane is acting from x = 0 to x = b. Identify the graph showing the variation of different quantities with distance.
A coil of effective area 4 m2 is placed at right angles to the magnetic induction B. The e.m.f. of 0.32 V is induced in the coil. When the field is reduced to 20% of its initial value in 0.5 sec. Find B (in wb/m2).
How much charge in terms of Faraday is required for the reduction of 1 mol of Cu2+ to Cu?
