Advertisements
Advertisements
प्रश्न
A hemispherical bowl made of brass has inner diameter 10.5 cm. Find the cost of tin-plating it on the inside at the rate of ₹ 16 per 100 cm2.
`["Assume "pi=22/7]`
उत्तर
Inner radius (r) of hemispherical bowl = `(10.5/2) cm` = 5.25 cm
Surface area of hemispherical bowl = 2πr2
= `[2xx22/7xx(5.25)^2]cm^2`
= 173.25 cm2
Cost of tin-plating 100 cm2 area = ₹ 16
Cost of tin-plating 173.25 cm2 area = `₹ (16 xx 173.25)/100`
= ₹ 27.72
Therefore, the cost of tin-plating the inner side of the hemispherical bowl is ₹ 27.72.
APPEARS IN
संबंधित प्रश्न
A solid cone of radius 5 cm and height 8 cm is melted and made into small spheres of radius 0.5 cm. Find the number of spheres formed.
A spherical ball of lead has been melted and made into identical smaller balls with radius equal to half the radius of the original one. How many such balls can be made?
How many balls each of radius 1 cm can be made by melting a bigger ball whose diameter is 8 cm?
A hemi-spherical bowl has negligible thickness and the length of its circumference is 198 cm. Find the capacity of the bowl.
Find the maximum volume of a cone that can be carved out of a solid hemisphere of radius r cm.
What is the least number of solid metallic spheres, each of 6 cm diameter, that should be melted and recast to form a solid metal cone whose height is 45 cm and diameter 12 cm?
If a sphere of radius 2r has the same volume as that of a cone with circular base of radius r, then find the height of the cone.
The largest sphere is cut off from a cube of side 6 cm. The volume of the sphere will be
Find the radius of a sphere whose surface area is equal to the area of the circle of diameter 2.8 cm
The given figure shows the cross-section of a cone, a cylinder and a hemisphere all with the same diameter 10 cm and the other dimensions are as shown.
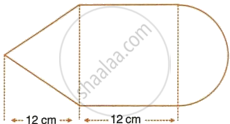
Calculate :
- the total surface area.
- the total volume of the solid and
- the density of the material if its total weight is 1.7 kg.
