Advertisements
Advertisements
प्रश्न
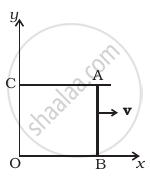
A magnetic field B = Bo sin ( ωt )`hatk` wire AB slides smoothly over two parallel conductors separated by a distance d (Figure). The wires are in the x-y plane. The wire AB (of length d) has resistance R and the parallel wires have negligible resistance. If AB is moving with velocity v, what is the current in the circuit. What is the force needed to keep the wire moving at constant velocity?
उत्तर
In this problem, the emf induced across AB is motional emf due to its motion, and emf induced by change in magnetic flux linked with the loop change due to a change of magnetic field.
In figure CA and OB are long parallel conducting wires, connected by d and conductor CO. The resistance of ACOB is negligible.
Let wire AB at t = 0 is at x = 0 i.e., on Y-axis.
Now AB moves with velocity `vhati`
Let at any time t, position of conductor AB is x(t) = `vhati t`
Motional e.m.f across AB
`V_(AB) = (W_(AB))/q = (D.d)/q = (qvi xx B)/q d`
`V_(AB) = vhati xx B_0 sin ωt. hatk xx d`
⇒ `e_1 = (B_0 sin ωt)vd (-hatj)`
And emf due to change in field (along OBAC)
`e_2 = (d(phi_B))/(dt)`
`phi_B = (B_0 sin ωt)(x(t)d)` ....(where, area A = xd)
`e_2 = - B_0 ω cos wtx(t)d`
Total emf in the circuit = emf due to change in field (along OBAC) + the emotional emf across AB
`e_1 + e_2 = - B_0d [ωx cos (t) + v sin (ωt)]`
The equivalent electrical diagram is shown in the diagram below.
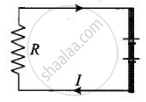
Electric current in clockwise direction is given by
= `(B_0d)/R (ωx cos ωt + v sin ωt)`
The force acting on the conductor is given by F = `ilB` sin 90° = `ilB`
Substituting the values,
`vecF_m = (B_0d)/R (ωx cos ωt + v sin ωt)(d)(B_0 sin ωt)(-hati)`
External force needed on wire is along positive x-axis to keep moving it with constant velocity is given by,
`vecF_(ext) = (B_0^2d^2)/R (ωx cos ωt + v sin ωt)sin ωt(hati)`
This is the required expression for force.
APPEARS IN
संबंधित प्रश्न
A conducting disc of radius r rotates with a small but constant angular velocity ω about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
A cycle wheel of radius 0.6 m is rotated with constant angular velocity of 15 rad/s in a region of magnetic field of 0.2 T which is perpendicular to the plane of the wheel. The e.m.f generated between its center and the rim is, ____________.
A straight conductor of length 2 m moves in a uniform magnetic field of induction 2.5 x `10^-3` T with a velocity. of 4 m/s in a direction perpendicular to its length and also perpendicular to the field. The e.m.f. induced between the ends of the conductor is ______.
The emf induced across the ends of a conductor due to its motion in a magnetic field is called motional emf. It is produced due to magnetic Lorentz force acting on the free electrons of the conductor. For a circuit shown in the figure, if a conductor of length l moves with velocity v in a magnetic field B perpendicular to both its length and the direction of the magnetic field, then all the induced parameters are possible in the circuit.
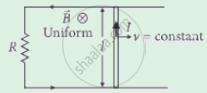
Direction of current induced in a wire moving in a magnetic field is found using ______.
The emf induced across the ends of a conductor due to its motion in a magnetic field is called motional emf. It is produced due to magnetic Lorentz force acting on the free electrons of the conductor. For a circuit shown in the figure, if a conductor of length l moves with velocity v in a magnetic field B perpendicular to both its length and the direction of the magnetic field, then all the induced parameters are possible in the circuit.

A bicycle generator creates 1.5 V at 15 km/hr. The EMF generated at 10 km/hr is ______.
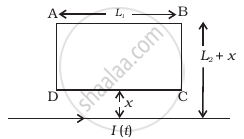
A rectangular loop of wire ABCD is kept close to an infinitely long wire carrying a current I(t) = Io (1 – t/T) for 0 ≤ t ≤ T and I(0) = 0 for t > T (Figure). Find the total charge passing through a given point in the loop, in time T. The resistance of the loop is R.
A simple pendulum with a bob of mass m and conducting wire of length L swings under gravity through an angle θ. The component of the earth's magnetic field in the direction perpendicular to the swing is B. Maximum emf induced across the pendulum is ______.
(g = acceleration due to gravity)
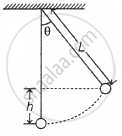
Derive an expression for the total emf induced in a conducting rotating rod.
A magnetic flux associated with a coil changes by 0.04 Wb in 0.2 second. The induced emf with coil is ______.
