Advertisements
Advertisements
प्रश्न
A conducting wire XY of mass m and neglibile resistance slides smoothly on two parallel conducting wires as shown in figure. The closed circuit has a resistance R due to AC. AB and CD are perfect conductors. There is a ˆ. magnetic field `B = B(t)hatk`.
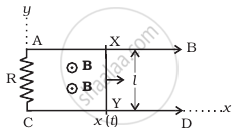
- Write down equation for the acceleration of the wire XY.
- If B is independent of time, obtain v(t) , assuming v(0) = u0.
- For (b), show that the decrease in kinetic energy of XY equals the heat lost in R.
उत्तर
First we have to analyse the situation as shown in the figure. Let the parallel wires be at y = 0 and y = L and are placed along x-axis. Wire XY is along y-axis.
At t = 0, wire AB starts from x = 0 and moves with a velocity v.
Let at time t, wire is at x(t) = vt. ....(Where x(t) is the displacement as a function of time).
Let us redraw the diagram as shown below.
(i) Let wire XY at t = 0 is at x = 0
And at t = t is at x = x(t)
Magnetic flux is a function of time `phi(t) = B(t) xx A`
∴ `phi(t) = B(t)l.x(t)`
`ε = - (dphi(t))/(dt) = - (dB(t))/(dt)l.x(t) - B(t)l. (dx(t))/(dt)`
`ε = (- dB(t))/(dt) l.x(t) - B(t)lv(t)`
The direction of induced current by Fleming's Right-Hand Rule or by Lenz's law is in clockwise direction in loop XY < AX.
`I = ε/R = (-l)/R [x(t) (dB(t))/(dt) + B(t)v(t)]` ......(I)
The force acting on the conductor is F = B(t) I l sin 90°
F = B(t)I.l
F = `(B(t)lε)/R = (-B(t)l^2)/R [(-dB(t))/(dt).x(t) - B(t).v(t)]`
`(md^2x)/(dt^2) = (-B(t)l^2)/R [x(t) (dB(t))/(dt) + B(t)v(t)]`
Or `(d^2x)/(dt^2) = (-l^2)/(mR) B(t) [x(t) (dB(t))/(dt) + B(t).v(t)]` ......(II)
(ii) Now B is independent of time i.e. B does not change with time or it is constant
∴ `(dB)/(dt)` = 0, B(t) = B and v(t) = c ......(III)
Put (III) in (II) we get
`(d^2x)/(dt^2) = (-l^2)/(mR) [0 + Bv]`
`(d^2x)/(dt^2) + (B^2l^2)/(mR) (dx)/(dt)` = 0
`(dv)/(dt) + (B^2l^2)/(mR) v` = 0
Integrating using variable separable from differential equation we have
`v = A exp((-l^2B^2t)/(mR))`
At t = 0, v = u
∴ `v(t) = u exp ((-l^2B^2t)/(mR))` ......(IV)
(iii) Heat lost per second in (ii) where `(dB)/(dt)` = 0
H = I2R
Magnitude of current from equation I in (i) part
`I = (Blv)/R = (-l)/R [0 + Bv]` ......`[because (dB)/(dt) = 0]`
Heat produced per second H = I2R
∴ `H = (B^2l^2v^2)/R^2 R`
`H = (B^2l^2)/R u^2 exp (-2i^2B^2t)/(mR)`
v from equation (IV) in (iii) part
Power lost = `int_0^t I^2 Rdt = (B^2l^2u^2)/R int_0^t e^((-2l^2B^2t)/(mR)) dt` ....`[because v^2 = u^2 exp ^((-2l^2B^2t)/(mR))]`
Power lost = `(B^2l^2u^2)/R (mR)/(2l^2B^2) [1 - e^((-2l^2B^2t)/(mR))]`
= `(m u^2)/2 [1 - e^((-2l^2B^2t)/(mR))]`
= `(m u^2)/2 - m/2 u^2e^((-2l^2B^2t)/(mR))`
= `[(m u^2)/2 - (mv^2(t))/2]`
= initial K.E. – final K.E.
Power lost = decrease in kinetic energy
This proves that decrease in K.E of XY is equal to the heat lost in R.
APPEARS IN
संबंधित प्रश्न
State Lenz's law. Illustrate, by giving an example, how this law helps in predicting the direction of the current in a loop in the presence of a changing magnetic flux.
Use Lenz’s law to determine the direction of induced current in the situation described by the figure:
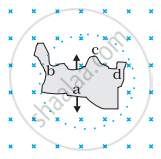
A wire of irregular shape turning into a circular shape.
The battery discussed in the previous question is suddenly disconnected. Is a current induced in the other loop? If yes, when does it start and when does it end? Do the loops attract each other or repel?
A pivoted aluminium bar falls much more slowly through a small region containing a magnetic field than a similar bar of an insulating material. Explain.
Two circular loops of equal radii are placed coaxially at some separation. The first is cut and a battery is inserted in between to drive a current in it. The current changes slightly because of the variation in resistance with temperature. During this period, the two loops _______________ .
Young's modulus for aluminium is 7 × 1010 Pa. The force needed to stretch an aluminium wire of diameter 2 mm and length 800 mm by 1 mm is ______.
Energy dissipate in LCR circuit in
Consider a metal ring kept (supported by a cardboard) on top of a fixed solenoid carrying a current I (Figure). The centre of the ring coincides with the axis of the solenoid. If the current in the solenoid is switched off, what will happen to the ring?

A metallic ring of mass m and radius `l` (ring being horizontal) is falling under gravity in a region having a magnetic field. If z is the vertical direction, the z-component of magnetic field is Bz = Bo (1 + λz). If R is the resistance of the ring and if the ring falls with a velocity v, find the energy lost in the resistance. If the ring has reached a constant velocity, use the conservation of energy to determine v in terms of m, B, λ and acceleration due to gravity g.
Predict the direction of induced current in the situation described by the following figure.