Advertisements
Advertisements
प्रश्न
A pin of length 2.0 cm lies along the principal axis of a converging lens, the centre being at a distance of 11 cm from the lens. The focal length of the lens is 6 cm. Find the size of the image.
उत्तर
Given,
Length of the pin = 2.0 cm
Focal length (f) of the lens = 6 cm
As per the question, the centre of the pin is 11 cm away from the lens.
i.e., the object distance (u) = 10 cm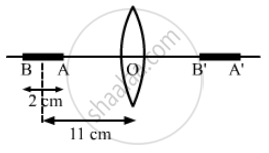
Since, we have to calculate the image of A and B, Let the image be A' and B'
So, the length of the A'B' = size of the image.
Using lens formula: \[\frac{1}{v_A} - \frac{1}{u_A} = \frac{1}{f}\]
Where vA and uA are the image and object distances from point A.
\[\Rightarrow \frac{1}{v_A} - \frac{1}{- 10} = \frac{1}{6}\]
\[\frac{1}{v_A} = \frac{1}{6} - \frac{1}{10} = \frac{1}{15}\]
\[v_A = 15 \text{ cm }\]
Similarly for point B,
Lens formula: \[\frac{1}{v_B} - \frac{1}{u_B} = \frac{1}{f}\]
Where vA and uA are the image and object distances from point B.
\[\frac{1}{v_B} - \frac{1}{- 12} = \frac{1}{6} \]
\[ \Rightarrow v_B = 12 \text{ cm }\]
Length of image = vA − vB = 15 − 12 = 3 cm.
APPEARS IN
संबंधित प्रश्न
A beam of light converges at a point P. Now a lens is placed in the path of the convergent beam 12 cm from P. At what point does the beam converge if the lens is
- a convex lens of focal length 20 cm, and
- a concave lens of focal length 16 cm?
An object of size 3.0 cm is placed 14 cm in front of a concave lens of focal length 21 cm. Describe the image produced by the lens. What happens if the object is moved further away from the lens?
You have learnt that plane and convex mirrors produce virtual images of objects. Can they produce real images under some circumstances? Explain.
A screen is placed 90 cm from an object. The image of the object on the screen is formed by a convex lens at two different locations separated by 20 cm. Determine the focal length of the lens.
An object 1.5 cm in size is placed on the side of the convex lens in the arrangement (a) above. The distance between the object and the convex lens is 40 cm. Determine the magnification produced by the two-lens system, and the size of the image
A card sheet divided into squares each of size 1 mm2 is being viewed at a distance of 9 cm through a magnifying glass (a converging lens of focal length 9 cm) held close to the eye.
- What is the magnification produced by the lens? How much is the area of each square in the virtual image?
- What is the angular magnification (magnifying power) of the lens?
- Is the magnification in (a) equal to the magnifying power in (b)? Explain.
An equiconvex lens of focal length 'f' is cut into two identical plane convex lenses. How will the power of each part be related to the focal length of the original lens ?
A double convex lens of + 5 D is made of glass of refractive index 1.55 with both faces of equal radii of curvature. Find the value of its radius of curvature.
Two converging lenses of unequal focal lengths can be used to reduce the aperture of a parallel beam of light without loosing the energy of the light. This increase the intensity. Describe how the converging lenses should be placed to do this.
Answer the following question.
An optical instrument uses a lens of 100 D for the objective lens and 50 D for its eyepiece. When the tube length is kept at 20 cm, the final image is formed at infinity.
(a) Identify the optical instrument.
(b) Calculate the magnification produced by the instrument.
Will the focal length of a lens for red light be more, same or less than that for blue light?
An unsymmetrical double convex thin lens forms the image of a point object on its axis. Will the position of the image change if the lens is reversed?
In many experimental set-ups the source and screen are fixed at a distance say D and the lens is movable. Show that there are two positions for the lens for which an image is formed on the screen. Find the distance between these points and the ratio of the image sizes for these two points.
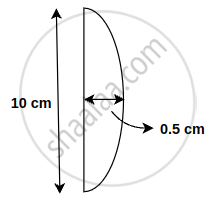
A plano convex lens has diameter of 10 cm and its thickness at the centre is 0.5 cm. Speed of light in the lens is 2 × 108 ms-1. What is the focal length of the lens?
In the given figure the radius of curvature of the curved face in the planoconvex and the planoconcave lens is 15 cm each. The refractive index of the material of the lenses is 1.5. Find the final position of the image formed.

