Advertisements
Advertisements
प्रश्न
An unsymmetrical double convex thin lens forms the image of a point object on its axis. Will the position of the image change if the lens is reversed?
उत्तर
Thin lens formula: `1/v - 1/u = 1/f`
For a given object position if focal length of the lens does not change, the image position remains unchanged.
By lens maker's formula,
`1/f = (mu - 1) (1/R_1 - 1/R_2)`
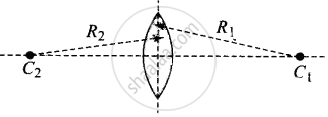
For this position R1 is positive
And R2 is negative. Hence focal length at this position
`1/f_1 = (mu - 1) (1/((+ R_1)) - 1/((-R_2))) = (mu - 1)(1/R_1 + 1/R_2)`
Now the lens is reversed,
At this position R2 is positive and R1 is negative. Hence focal length at this position is
`1/f_2 = (mu - 1) (1/((+ R_2)) - 1/((-R_1))) = (mu - 1)(1/R_1 + 1/R_2)`
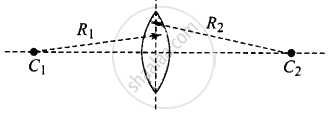
We can observe the focal length of the lens does not change in both positions, hence the image position remains unchanged.
APPEARS IN
संबंधित प्रश्न
You have learnt that plane and convex mirrors produce virtual images of objects. Can they produce real images under some circumstances? Explain.
The image of a small electric bulb fixed on the wall of a room is to be obtained on the opposite wall 3 m away by means of a large convex lens. What is the maximum possible focal length of the lens required for the purpose?
A screen is placed 90 cm from an object. The image of the object on the screen is formed by a convex lens at two different locations separated by 20 cm. Determine the focal length of the lens.
- Determine the ‘effective focal length’ of the combination of the two lenses, if they are placed 8.0 cm apart with their principal axes coincident. Does the answer depend on which side of the combination a beam of parallel light is incident? Is the notion of the effective focal length of this system useful at all?
- An object 1.5 cm in size is placed on the side of the convex lens in the arrangement (a) above. The distance between the object and the convex lens is 40 cm. Determine the magnification produced by the two-lens system and the size of the image.
An object 1.5 cm in size is placed on the side of the convex lens in the arrangement (a) above. The distance between the object and the convex lens is 40 cm. Determine the magnification produced by the two-lens system, and the size of the image
A man with normal near point (25 cm) reads a book with small print using a magnifying glass: a thin convex lens of focal length 5 cm.
(a) What is the closest and the farthest distance at which he should keep the lens from the page so that he can read the book when viewing through the magnifying glass?
(b) What is the maximum and the minimum angular magnification (magnifying power) possible using the above simple microscope?
Two concave lenses L1 and L2 are kept in contact with each other. If the space between the two lenses is filled with a material of smaller refractive index, the magnitude of the focal length of the combination
A pin of length 2.0 cm lies along the principal axis of a converging lens, the centre being at a distance of 11 cm from the lens. The focal length of the lens is 6 cm. Find the size of the image.
Will the focal length of a lens for red light be more, same or less than that for blue light?
In many experimental set-ups the source and screen are fixed at a distance say D and the lens is movable. Show that there are two positions for the lens for which an image is formed on the screen. Find the distance between these points and the ratio of the image sizes for these two points.
