Advertisements
Advertisements
प्रश्न
A ray PQ incident on the face AB of a prism ABC, as shown in the figure, emerges from the face AC such that AQ = AR.
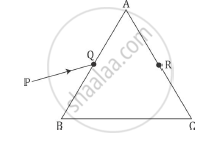
Draw the ray diagram showing the passage of the ray through the prism. If the angle of the prism is 60° and refractive index of the material of prism is `sqrt3` , determine the values of angle of incidence and angle of deviation
उत्तर
Given that side AQ = AR. This implies that ∠AQR = ∠ ARQ.
The ray diagram for the refraction of ray PQ passing through the prism ABC is as shown below.

As the ray PQ after refraction from surface AB emerges from face AC at point R of the prism, it implies that the refracted ray QR travels parallel to the base of the prism. This happens at the minimum deviation position. So, according to the angle of minimum deviation formula, we have
`mu=(sin(A+delta_m)/2)/(sinA/2)" .......1"`
where A is the angle of prism δmis the angle of minimum deviation and μ is the refractive index of the prism.
Given A =60° , n = `sqrt3`
Substituting in (1) we get:
`sqrt3=(sin""(60^@+delta_m)/2)/(sin60^@/2)`
`sqrt3xxsin30^@=sin""((60^@+delta_m))/2`
`sqrt3/2=sin"" ((60^@+delta_m))/2`
`sin^(-1)(sqrt3/2)=((60^@+delta_m))/2`
`60^@=((60^@+delta_m))/2`
120° = (60°+δm)
⇒ δm = 60°
Thus, the angle of minimum deviation = 60°.
At the minimum deviation position,
`i=(A+delta_m)/2`
We know A = 60°, δm = 60°
Substituting we get:
`i=(60^@+60^@)/2`
⇒ i = 60°
APPEARS IN
संबंधित प्रश्न
A ray PQ incident normally on the refracting face BA is refracted in the prism BAC made of material of refractive index 1.5. Complete the path of ray through the prism. From which face will the ray emerge? Justify your answer.
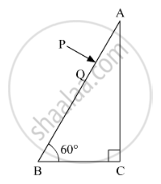
A ray of light passes through an equilateral glass prism such that the angle of incidence is equal to the angle of emergence and each of these angles is equal to 3/4 of angle of prism. Find the angle of deviation.
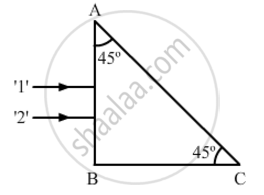
Two monochromatic rays of light are incident normally on the face AB of an isosceles right-angled prism ABC. The refractive indices of the glass prism for the two rays '1' and '2' are respectively 1.38 and 1.52. Trace the path of these rays after entering through the prism.
The angular dispersion produced by a prism ___________ .
A prism can produce a minimum deviation δ in a light beam. If three such prisms are combined, the minimum deviation that can be produced in this beam is _______________.
Two prisms of identical geometrical shape are combined with their refracting angles oppositely directed. The materials of the prisms have refractive indices 1.52 and 1.62 for violet light. A violet ray is deviated by 1.0° when passes symmetrically through this combination. What is the angle of the prisms?
Three thin prisms are combined as shown in figure. The refractive indices of the crown glass for red, yellow and violet rays are μr, μy and μv respectively and those for the flint glass are μ'r, μ'y and μ'v respectively. Find the ratio A'/A for which (a) there is no net angular dispersion, and (b) there is no net deviation in the yellow ray.
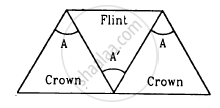
A thin prism of crown glass (μr = 1.515, μv = 1.525) and a thin prism of flint glass (μr = 1.612, μv = 1.632) are placed in contact with each other. Their refracting angles are 5.0° each and are similarly directed. Calculate the angular dispersion produced by the combination.
In a regular prism, what is the relation between angle of incidence and angle of emergence when it is in the minimum deviation position?
For any prism, obtain a relation between the angle of the prism (A), the angle of minimum deviation (δm) and the refractive index of its material (μ or n).
