Advertisements
Advertisements
Question
A ray PQ incident on the face AB of a prism ABC, as shown in the figure, emerges from the face AC such that AQ = AR.
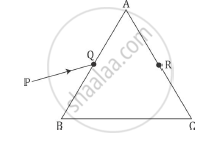
Draw the ray diagram showing the passage of the ray through the prism. If the angle of the prism is 60° and refractive index of the material of prism is `sqrt3` , determine the values of angle of incidence and angle of deviation
Solution
Given that side AQ = AR. This implies that ∠AQR = ∠ ARQ.
The ray diagram for the refraction of ray PQ passing through the prism ABC is as shown below.

As the ray PQ after refraction from surface AB emerges from face AC at point R of the prism, it implies that the refracted ray QR travels parallel to the base of the prism. This happens at the minimum deviation position. So, according to the angle of minimum deviation formula, we have
`mu=(sin(A+delta_m)/2)/(sinA/2)" .......1"`
where A is the angle of prism δmis the angle of minimum deviation and μ is the refractive index of the prism.
Given A =60° , n = `sqrt3`
Substituting in (1) we get:
`sqrt3=(sin""(60^@+delta_m)/2)/(sin60^@/2)`
`sqrt3xxsin30^@=sin""((60^@+delta_m))/2`
`sqrt3/2=sin"" ((60^@+delta_m))/2`
`sin^(-1)(sqrt3/2)=((60^@+delta_m))/2`
`60^@=((60^@+delta_m))/2`
120° = (60°+δm)
⇒ δm = 60°
Thus, the angle of minimum deviation = 60°.
At the minimum deviation position,
`i=(A+delta_m)/2`
We know A = 60°, δm = 60°
Substituting we get:
`i=(60^@+60^@)/2`
⇒ i = 60°
APPEARS IN
RELATED QUESTIONS
Plot a graph to show the variation of the angle of deviation as a function of the angle of incidence for light passing through a prism. Derive an expression for the refractive index of the prism in terms of angle of minimum deviation and angle of the prism.
A ray PQ incident on the refracting face BA is refracted in the prism BAC as shown in the figure and emerges from the other refracting face AC as RS such that AQ = AR. If the angle of prism A = 60° and refractive index of material of prism is `sqrt3 `. Calculate angle θ.

A ray of light incident normally on one face of a right isosceles prism is totally reflected, as shown in fig. What must be the minimum value of refractive index of glass? Give relevant calculations.
Draw the ray diagram showing refraction of light through a glass prism and hence obtain the relation between the refractive index μ of the prism, angle of prism and angle of minimum deviation.
Out of blue and red light which is deviated more by a prism? Give reason.
If three identical prisms are combined, is it possible to pass a beam that emerges undeviated? Undispersed?
A thin prism is made of a material having refractive indices 1.61 and 1.65 for red and violet light. The dispersive power of the material is 0.07. It is found that a beam of yellow light passing through the prism suffers a minimum deviation of 4.0° in favourable conditions. Calculate the angle of the prism.
A narrow beam of monochromatic light, PQ, is incident normally on one face of an equiangular glass prism of refractive index 1.45. When the prism is immersed in a certain liquid, the ray makes a grazing emergence along the other face (See figure). Find the refractive index of this liquid. 
An equilateral glass prism has a refractive index 1.6 in the air. Calculate the angle of minimum deviation of the prism, when kept in a medium of refractive index `4sqrt(2)"/"5.`
Define angular dispersion.
