Advertisements
Advertisements
प्रश्न
A small flat search coil of area 5cm2 with 140 closely wound turns is placed between the poles of a powerful magnet producing magnetic field 0.09T and then quickly removed out of the field region. Calculate:
(a) Change of magnetic flux through the coil, and
(b) emf induced in the coil.
उत्तर १
a = 5cm2
= 5 × 10-4 m2
n = 140
(a) B = 0.09T
`Φ = bar(B) . bar(A) = BA cos theta = 0.09 xx 5 xx10^(-4) xx 1`
= 0.45 × 10-4 W b
(b) `e = n (d phi )/(dt)`
= `140 xx (0.45 xx 10^(-4))/dt`
but dt is very small
dt → 0
`therefore -> ∞`
उत्तर २
Number of turns in the coil, N = 140
Area of cross-section of the coil = 5 cm2
The magnetic field, B = 0.09 T
(a) Flux linked with the coil, Φ = NBA cos θ
θ = 0° , Φi = NBA
⇒ Φi = 140 × 0.09 × 5 × 10-4
⇒ Φi = 6.3 × 10-3 Weber
When the coil is quickly removed, the flux linkage becomes zero,Φf = 0
Hence change in magnetic flux through the coil,
ΔΦ = Φf -Φi = (0 - 6.3 × 10-3) Weber
ΔΦ = - 6.3 × 10-3 Weber
(b) Let the coil is removed in a very short period of time-interval, dt = 1s, then induced emf,
`"e" = -("d"phi)/("dt") = - ((-6.3xx10^-3)/1) = 6.3 xx 10^-3 "V"`
संबंधित प्रश्न
Two cells of emf E1 and E2 and internal resistances r1 and r2 are connected in parallel. Derive the expression for the (i) emf and (ii) internal resistance of a single equivalent cell which can replace this combination.
State Lenz’s Law.
A metallic rod held horizontally along east-west direction, is allowed to fall under gravity. Will there be an emf induced at its ends? Justify your answer.
A metallic rod of ‘L’ length is rotated with angular frequency of ‘ω’ with one end hinged at the centre and the other end at the circumference of a circular metallic ring of radius L, about an axis passing through the centre and perpendicular to the plane of the ring. A constant and uniform magnetic field B parallel to the axis is presents everywhere. Deduce the expression for the emf between the centre and the metallic ring.
A conducting circular loop having a radius of 5.0 cm, is placed perpendicular to a magnetic field of 0.50 T. It is removed from the field in 0.50 s. Find the average emf produced in the loop during this time.
A wire-loop confined in a plane is rotated in its own plane with some angular velocity. A uniform magnetic field exists in the region. Find the emf induced in the loop.
A wire of length 10 cm translates in a direction making an angle of 60° with its length. The plane of motion is perpendicular to a uniform magnetic field of 1.0 T that exists in the space. Find the emf induced between the ends of the rod if the speed of translation is 20 cm s−1.
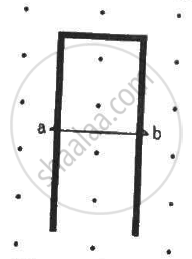
A conducting wire ab of length l, resistance r and mass m starts sliding at t = 0 down a smooth, vertical, thick pair of connected rails as shown in figure. A uniform magnetic field B exists in the space in a direction perpendicular to the plane of the rails. (a) Write the induced emf in the loop at an instant t when the speed of the wire is v. (b) What would be the magnitude and direction of the induced current in the wire? (c) Find the downward acceleration of the wire at this instant. (d) After sufficient time, the wire starts moving with a constant velocity. Find this velocity vm. (e) Find the velocity of the wire as a function of time. (f) Find the displacement of the wire as a function of time. (g) Show that the rate of heat developed in the wire is equal to the rate at which the gravitational potential energy is decreased after steady state is reached.
A bicycle is resting on its stand in the east-west direction and the rear wheel is rotated at an angular speed of 100 revolutions per minute. If the length of each spoke is 30.0 cm and the horizontal component of the earth's magnetic field is 2.0 × 10−5 T, find the emf induced between the axis and the outer end of a spoke. Neglect centripetal force acting on the free electrons of the spoke.
Two identical coaxial coils P and Q carrying equal amount of current in the same direction are brought nearer. The current in ______.
