Advertisements
Advertisements
प्रश्न
A train, standing in a station-yard, blows a whistle of frequency 400 Hz in still air. The wind starts blowing in the direction from the yard to the station with at a speed of 10 m s–1. What are the frequency, wavelength, and speed of sound for an observer standing on the station’s platform? Is the situation exactly identical to the case when the air is still and the observer runs towards the yard at a speed of 10 m s–1? The speed of sound in still air can be taken as 340 m s–1.
Advertisements
उत्तर १
The travelling harmonic wave is y(x, t) = `7.5 sin (0.0050x+ 12t + pi/4)`
At x = 1 cm and t = 1 sec
`y (1, 1) = 7.5 sin (0.005 xx 1 + 12 xx 1 pi/4) = 7.5 sin (12.005 + pi/4)` ....(i)
Now `theta = (12.005 + pi/4) "radian"`
`= 180/pi (12.005 + pi/4) "degree" = (12.005 xx 180)/(22/7) + 45 = 732.55^@`
`:. From (i), y(1,2) = 7.5 sin (732.55^@) = 7.5 sin (720 + 12.55^@)`
`= 7.5 sin 12.55^@ = 7.5 xx 0.2173 = 1.63 cm`
Velocity of oscillation `v = (dy)/(dt) (1,1) = d/dt[7.5 sin(0.005x + 12 + pi/4)]`
`= 7.5 xx 12 cos [0.005x + 12t + pi/4]`
At x = 1 cm, t = 1 sec
`v = 7.5 xx 12 cos (0.005 + 12 + pi/4) = 90 cos (732.35^@)`
= 90 cos (720 + 12.55)
`v = 90 cos (12.55^@) = 90 xx 0.9765 = 87.89 "cm/s"`
Comparing the given equation with the standard from `y(x,t)= t sin[pi/4 (vt + x) + phi_0]`
We get `r = 7.5 cm, (2piv)/lambda = 12 or 2 piv = 12 `
`v = 6/pi`
`(2pi)/lambda` = 0.005
`:. lambda = 2pi/0.005 = (2xx3.14)/0.005 = 1256 cm = 12.56 m`
Velocity of wave propagation, `v = vlambda = 6/pi xx 12.56 = 24 "m/s"`
We find that velocity at x = 1 cm, t = 1 sec is not equal tovelocity of waves propagation.
b) Now all point which are at a distance of `+- lambda +-2lambda, +- 3lambda` from x = 1 cm will have same tranverse displacement and velocity.As lambda = 12.56 m, therefore all points at distances `+- 12.6 m, +- 25.2 m, +- 37.8 m` from x = 1 cm will have same dispalcement and velocity as at x=1 point t =2 s, 5s and 11s
उत्तर २
For the stationary observer: 400 Hz; 0.875 m; 350 m/s
For the running observer: Not exactly identical
For the stationary observer:
Frequency of the sound produced by the whistle, ν = 400 Hz
Speed of sound = 340 m/s
Velocity of the wind, v = 10 m/s
As there is no relative motion between the source and the observer, the frequency of the sound heard by the observer will be the same as that produced by the source, i.e., 400 Hz.
The wind is blowing toward the observer. Hence, the effective speed of the sound increases by 10 units, i.e.,
Effective speed of the sound, ve = 340 + 10 = 350 m/s
The wavelength (λ) of the sound heard by the observer is given by the relation:
`lambda = V_e/V` = 350/400 = 0.875 m`
For the running observer:
Velocity of the observer, vo = 10 m/s
The observer is moving toward the source. As a result of the relative motions of the source and the observer, there is a change in frequency (v').
This is given by the relation:
`v' = ((v + v_0)/v)v`
`= ((340+ 10)/340) xx 400 = 411.76 "Hz"`
Since the air is still, the effective speed of sound = 340 + 0 = 340 m/s
The source is at rest. Hence, the wavelength of the sound will not change, i.e., λ remains 0.875 m.
Hence, the given two situations are not exactly identical.
APPEARS IN
संबंधित प्रश्न
A steel wire has a length of 12.0 m and a mass of 2.10 kg. What should be the tension in the wire so that speed of a transverse wave on the wire equals the speed of sound in dry air at 20 °C = 343 m s–1.
You have learnt that a travelling wave in one dimension is represented by a function y= f (x, t)where x and t must appear in the combination x – v t or x + v t, i.e. y = f (x ± v t). Is the converse true? Examine if the following functions for y can possibly represent a travelling wave:
(a) `(x – vt )^2`
(b) `log [(x + vt)/x_0]`
(c) `1/(x + vt)`
A metre-long tube open at one end, with a movable piston at the other end, shows resonance with a fixed frequency source (a tuning fork of frequency 340 Hz) when the tube length is 25.5 cm or 79.3 cm. Estimate the speed of sound in air at the temperature of the experiment. The edge effects may be neglected.
Show that for a wave travelling on a string
\[\frac{y_{max}}{\nu_{max}} = \frac{\nu_{max}}{\alpha_{max}},\]
where the symbols have usual meanings. Can we use componendo and dividendo taught in algebra to write
\[\frac{y_{max} + \nu_{max}}{\nu_{max} - \nu_{max}} = \frac{\nu_{max} + \alpha_{max}}{\nu_{max} - \alpha_{max}}?\]
Two waves of equal amplitude A, and equal frequency travel in the same direction in a medium. The amplitude of the resultant wave is
The displacement of the particle at x = 0 of a stretched string carrying a wave in the positive x-direction is given f(t) = A sin (t/T). The wave speed is v. Write the wave equation.
A wave pulse is travelling on a string with a speed \[\nu\] towards the positive X-axis. The shape of the string at t = 0 is given by g(x) = Asin(x/a), where A and a are constants. (a) What are the dimensions of A and a ? (b) Write the equation of the wave for a general time t, if the wave speed is \[\nu\].
A wave propagates on a string in the positive x-direction at a velocity \[\nu\] \[t = t_0\] is given by \[g\left( x, t_0 \right) = A \sin \left( x/a \right)\]. Write the wave equation for a general time t.
A wave travelling on a string at a speed of 10 m s−1 causes each particle of the string to oscillate with a time period of 20 ms. (a) What is the wavelength of the wave? (b) If the displacement of a particle of 1⋅5 mm at a certain instant, what will be the displacement of a particle 10 cm away from it at the same instant?
A string of length 40 cm and weighing 10 g is attached to a spring at one end and to a fixed wall at the other end. The spring has a spring constant of 160 N m−1 and is stretched by 1⋅0 cm. If a wave pulse is produced on the string near the wall, how much time will it take to reach the spring?
A 200 Hz wave with amplitude 1 mm travels on a long string of linear mass density 6 g m−1 kept under a tension of 60 N. (a) Find the average power transmitted across a given point on the string. (b) Find the total energy associated with the wave in a 2⋅0 m long portion of the string.
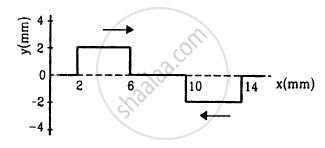
Following figure shows two wave pulses at t = 0 travelling on a string in opposite directions with the same wave speed 50 cm s−1. Sketch the shape of the string at t = 4 ms, 6 ms, 8 ms, and 12 ms.
A steel wire fixed at both ends has a fundamental frequency of 200 Hz. A person can hear sound of maximum frequency 14 kHz. What is the highest harmonic that can be played on this string which is audible to the person?
Figure shows an aluminium wire of length 60 cm joined to a steel wire of length 80 cm and stretched between two fixed supports. The tension produced is 40 N. The cross-sectional area of the steel wire is 1⋅0 mm2 and that of the aluminium wire is 3⋅0 mm2. What could be the minimum frequency of a tuning fork which can produce standing waves in the system with the joint as a node? The density of aluminium is 2⋅6 g cm−3 and that of steel is 7⋅8 g cm−3.
Following figure shows a string stretched by a block going over a pulley. The string vibrates in its tenth harmonic in unison with a particular tuning for. When a beaker containing water is brought under the block so that the block is completely dipped into the beaker, the string vibrates in its eleventh harmonic. Find the density of the material of the block.
A man standing unsymmetrical position between two mountains and fires a gun. He hears the first echo after 1.5 s and the second echo after 2.5 s. If the speed of sound in air is 340 m/s, then the distance between the mountains will be ______
An organ pipe of length 0.4 m is open at both ends. The speed of sound in the air is 340 m/s. The fundamental frequency is ______
A transverse harmonic wave on a string is described by y(x, t) = 3.0 sin (36t + 0.018x + π/4) where x and y are in cm and t is in s. The positive direction of x is from left to right.
- The wave is travelling from right to left.
- The speed of the wave is 20 m/s.
- Frequency of the wave is 5.7 Hz.
- The least distance between two successive crests in the wave is 2.5 cm.
Speed of sound waves in a fluid depends upon ______.
- directty on density of the medium.
- square of Bulk modulus of the medium.
- inversly on the square root of density.
- directly on the square root of bulk modulus of the medium.
A wave of frequency υ = 1000 Hz, propagates at a velocity v = 700 m/sec along x-axis. Phase difference at a given point x during a time interval M = 0.5 × 10-3 sec is ______.
