Advertisements
Advertisements
प्रश्न
ABCD is a square. The cooordinates of B and D are (-3, 7) and (5, -1) respectively. Find the equation of AC.
उत्तर
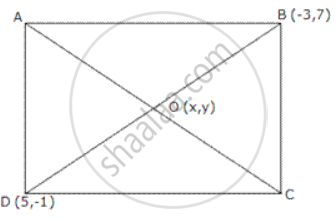
Slope of BD = `(7 + 1)/(-3 -5)` = 1
Slope of AC = 1
Mid point of AC = mid point of BD
O(x,y) = `((5 - 3)/2 , (-1 + 7)/2)` = (1,3)
Equation of AC ⇒ `("y" - "y"_1)/("x" - "x"_1)` = slope
⇒ `("y" - 3)/("x" - 1) = 1`
⇒ x - 1 = y - 3
⇒ x - y + 2 = 0
⇒ y = x + 2
APPEARS IN
संबंधित प्रश्न
The equation of a line 3x + 4y − 7 = 0. Find
1) The slope of the line.
2) The equation of a line perpendicular to the given line and passing through the intersection of the lines x – y + 2 = 0 and 3x + y – 10 = 0.
The point (−3, 2) lies on the line ax + 3y + 6 = 0, calculate the value of a.
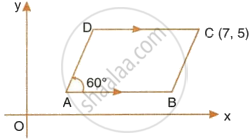
The following figure shows a parallelogram ABCD whose side AB is parallel to the x-axis, ∠A = 60° and vertex C = (7, 5). Find the equations of BC and CD.
The line through P (5, 3) intersects y-axis at Q.
write the equation of the line
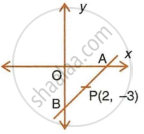
A and B are two points on the x-axis and y-axis respectively. P(2, −3) is the mid point of AB. Find the
- co-ordinates of A and B
- slope of line AB
- equation of line AB.
Find the equation of a line whose slope and y-intercept are m = `(-6)/5`, c = 3
ABCD is rhombus. The coordinates of A and C ae (3,7) and (9,15). Find the equation of BD.
Find the equation of a straight line which cuts an intercept of 5 units on Y-axis and is parallel to the line joining the points (3, – 2) and (1, 4).
Find the equation of the line passing through (0, 4) and parallel to the line 3x + 5y + 15 = 0.
Find the equation of the straight line which has Y-intercept equal to 4/3 and is perpendicular to 3x – 4y + 11 = 0.
