Advertisements
Advertisements
प्रश्न
Calculate the focal length of a corrective lens having power +2.5 D.
उत्तर
Solution: Data: Convex lens, P = +2.5 D, f = ?
P = 1/f
∴ 2.5 D = 1/f
∴ f = 1/2.5D = 0.4m = 40cm
The focal length of the lens is 40 cm.
APPEARS IN
संबंधित प्रश्न
Calculate the focal length of a corrective lens having power +2D.
(a) At what distance should the lens be held from the figure in order to view the squares distinctly with the maximum possible magnifying power?
(b) What is the magnification in this case?
(c) Is the magnification equal to the magnifying power in this case? Explain.

The lens A has a focal length of 25 cm whereas another lens B has a focal length of 60 cm. Giving reason state, which lens has more power: A or B.
The power of a lens is + 0.2 D. Calculate its focal length.
The power of a lens is, −2 D. What is its focal length?
A doctor has prescribed a corrective lens of power, −1.5 D. Find the focal length of the lens. Is the prescribed lens diverging or converging?
A lens has a focal length of, −10 cm. What is the power of the lens and what is its nature?
A convex lens of focal length 25 cm and a concave lens of focal length 10 cm are placed in close contact with one another.
(a) What is the power of this combination?
(b) What is the focal length of this combination?
(c) Is this combination converging or diverging?
The power of a combination of two lenses X and Y is 5 D. If the focal length of lens X be 15 cm :
(a) calculate the focal length of lens Y.
(b) state the nature of lens Y.
Two lenses A and B have focal lengths of +20 cm and, −10 cm, respectively.
(a) What is the nature of lens A and lens B?
(b) What is the power of lens A and lens B?
(c) What is the power of combination if lenses A and B are held close together?
A diverging lens has a focal length of 0.10 m. The power of this lens will be:
The power of a lens is +2.0D. Its focal length should be :
On reducing the focal length of a lens, its power ______.
Find the radius of curvature of the convex surface of a plano-convex lens, whose focal length is 0.3 m and the refractive index of the material of the lens is 1.5.
A symmetric double convex lens is cut in two equal parts by a plane containing the principal axis. If the power of the original lens was 4 D, the power of a divided lens will be
A pin of length 2.00 cm is placed perpendicular to the principal axis of a converging lens. An inverted image of size 1.00 cm is formed at a distance of 40.0 cm from the pin. Find the focal length of the lens and its distance from the pin.
A 5.0 diopter lens forms a virtual image which is 4 times the object placed perpendicularly on the principal axis of the lens. Find the distance of the object from the lens.
How is accommodation produced?
Complete the diagram to show the formation of the image of the object AB.
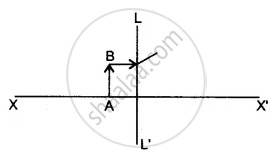
(i) Name the Lens LL’.
(ii) Where is the image of the object AB formed?
(iii) State three characteristics of the image.
A convex lens is of focal length 20 cm. Find its power.
The power of the magnifying glass depends on the distance of the magnifying glass from object.
Find the power of a convex lens of focal length of + 25 cm.
Assertion and reasoning type
- Assertion: Myopia is due to the increase in the converging power of eye lens.
- Reason: Myopia can be corrected with the help of concave lens.
The focal length of a concave lens is 20 cm. The focal length of a convex lens is 25 cm. These two are placed in contact with each other. What is the power of the combination? Is it diverging, converging or undeviating in nature?
A convex lens with radii of curvature R1 = R2 is immersed in water. Assuming that the refractive indices of glass and water are 3/2 and 4/3 respectively, its focal length f1 in comparison to that in air, f, is ______.
The same size images are formed by a convex lens when the object is placed at 20 cm or at 10 cm from the lens. The focal length of convex lens is ______ cm.
When monochromatic red light is used instead of blue light in a convex lens, its focal length will ______.
An object is kept at a distance of 1m from a lens of power +2D:
- Identify the type of lens.
- Calculate its focal length and distance of the image formed.
The focal length of a double convex lens is equal to the radius of curvature of either surface. What is the refractive index of its material?
In what unit is the power of a lens expressed?
