Advertisements
Advertisements
प्रश्न
Construct a triangle ABC in which angle ABC = 75°, AB = 5 cm and BC = 6.4 cm. Draw perpendicular bisector of side BC and also the bisector of angle ACB. If these bisectors intersect each other at point P; prove that P is equidistant from B and C; and also from AC and BC.
उत्तर
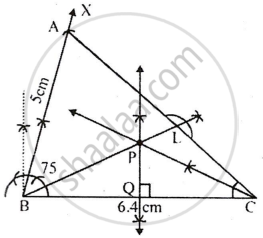
Steps of Construction:
- Draw a line segment BC = 6.4 cm
- At B, draw a ray BX making an angle of 75° with BC and cut off BA = 5 cm.
- Join AC. ΔABC is the required triangle.
- Draw the perpendicular bisector of BC.
- Draw the angle bisector of angle ACB which intersects the perpendicular bisector of BC at P.
- Join PB and draw PL ⊥ AC.
Proof: In ΔPBQ and ΔPCQ
PQ = PQ ...(Common)
∠AQB = ∠PQC ...(Each = 90°)
BQ = QC ...(PQ is the perpendicular bisector of BC)
∴ By side Angle side criterion of congruence
ΔPBQ ≅ ΔPCQ ...(SAS Postulate)
The corresponding parts of the congruent triangle are congruent
∴ PB = PC ...(C.P.C.T.)
Hence, P is equidistant from B and C.
∠PQC = ∠PLC ...(Each = 90°)
∠PCQ = ∠PCL ...(Given)
PC = PC ...(Common)
Again in ΔPQC and ΔPLC
∴ By Angle – Angle side criterion of congruence,
ΔPQC ≅ ΔPLC ...(AAS postulate)
The corresponding parts of the congruent triangles are congruent
∴ PQ = PL ...(C.P.C.T.)
Hence, P is equidistant from AC and BC.
APPEARS IN
संबंधित प्रश्न
Given: PQ is perpendicular bisector of side AB of the triangle ABC.

Prove: Q is equidistant from A and B.
Given: CP is bisector of angle C of ΔABC.
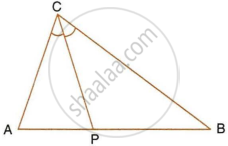
Prove: P is equidistant from AC and BC.
Given: AX bisects angle BAC and PQ is perpendicular bisector of AC which meets AX at point Y.
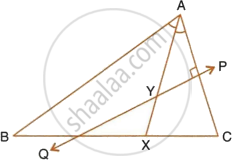
Prove:
- X is equidistant from AB and AC.
- Y is equidistant from A and C.
Describe the locus of the centre of a given circle which rolls around the outside of a second circle and is always touching it.
Describe the locus of the centres of all circles that are tangent to both the arms of a given angle.
Describe the locus of the mid-points of all chords parallel to a given chord of a circle.
Describe the locus of points within a circle that are equidistant from the end points of a given chord.
Construct a triangle ABC, having given AB = 4.8 cm, AC = 4 cm, and ∠A = 75°. Find a point P.
- inside the triangle ABC.
- outside the triangle ABC
Equidistant from B and C; and at a distance of 1.2 cm from BC.
