Advertisements
Advertisements
प्रश्न
Construct a right angled triangle PQR, in which ∠Q = 90°, hypotenuse PR = 8 cm and QR = 4.5 cm. Draw bisector of angle PQR and let it meets PR at point T. Prove that T is equidistant from PQ and QR.
उत्तर

Steps of Construction:
- Draw a line segment QR = 4.5 cm
- At Q, draw a ray QX making an angle of 90°
- With centre R and radius 8 cm, draw an arc which intersects QX at P.
- Join RP. ΔPQR is the required triangle.
- Draw the bisector of ∠PQR that meets PR in T.
- From T, draw perpendicular PL and PM respectively on PQ and QR.
Proof: In ΔLTQ and ΔMTQ
∠TLQ = ∠TMQ ...(Each = 90°)
∠LQT = ∠TQM ...(QT is angle bisector)
QT = QT ...(Common)
∴ By Angle – Angle – side criterion of congruence,
∴ ΔLTQ ≅ ΔMTQ ...(AAS postulate)
The corresponding parts of the congruent triangles are congruent
∴ TL = TM ...(C.P.C.T.)
Hence, T is equidistant from PQ and QR.
APPEARS IN
संबंधित प्रश्न
In parallelogram ABCD, side AB is greater than side BC and P is a point in AC such that PB bisects angle B. Prove that P is equidistant from AB and BC.
In triangle LMN, bisectors of interior angles at L and N intersect each other at point A. Prove that:
- Point A is equidistant from all the three sides of the triangle.
- AM bisects angle LMN.
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
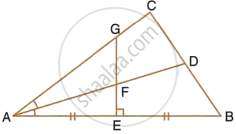
F is equidistant from A and B.
The given figure shows a triangle ABC in which AD bisects angle BAC. EG is perpendicular bisector of side AB which intersects AD at point F.
Prove that:
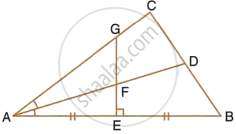
F is equidistant from AB and AC.
The bisectors of ∠B and ∠C of a quadrilateral ABCD intersect each other at point P. Show that P is equidistant from the opposite sides AB and CD.
Describe the locus of a point in rhombus ABCD, so that it is equidistant from
- AB and BC;
- B and D.
In a quadrilateral PQRS, if the bisectors of ∠ SPQ and ∠ PQR meet at O, prove that O is equidistant from PS and QR.
Find the locus of the centre of a circle of radius r touching externally a circle of radius R.
ΔPBC and ΔQBC are two isosceles triangles on the same base BC but on the opposite sides of line BC. Show that PQ bisects BC at right angles.
Given: ∠BAC, a line intersects the arms of ∠BAC in P and Q. How will you locate a point on line segment PQ, which is equidistant from AB and AC? Does such a point always exist?
