Advertisements
Advertisements
प्रश्न
In each of the given figures; PA = PB and QA = QB.
| i. | 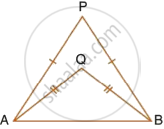 |
| ii. | 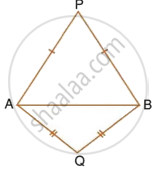 |
Prove, in each case, that PQ (produce, if required) is perpendicular bisector of AB. Hence, state the locus of the points equidistant from two given fixed points.
उत्तर
Construction: Join PQ which meets AB in D.
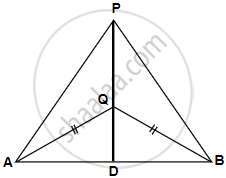
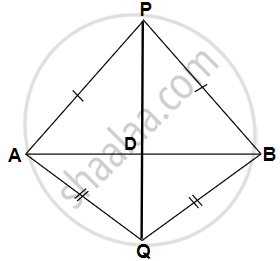
Proof: P is equidistant from A and B.
∴ P lies on the perpendicular bisector of AB.
Similarly, Q is equidistant from A and B.
∴ Q lies on perpendicular bisector of AB.
∴ P and Q both lie on the perpendicular bisector of AB.
∴ PQ is perpendicular bisector of AB.
Hence, locus of the points which are equidistant from two fixed points, is a perpendicular bisector of the line joining the fixed points.
APPEARS IN
संबंधित प्रश्न
The bisectors of ∠B and ∠C of a quadrilateral ABCD intersect each other at point P. Show that P is equidistant from the opposite sides AB and CD.
Draw a line AB = 6 cm. Draw the locus of all the points which are equidistant from A and B.
In the figure given below, find a point P on CD equidistant from points A and B.

Describe the locus of the centres of all circles passing through two fixed points.
A straight line AB is 8 cm long. Draw and describe the locus of a point which is:
- always 4 cm from the line AB.
- equidistant from A and B.
Mark the two points X and Y, which are 4 cm from AB and equidistant from A and B. Describe the figure AXBY.
In a quadrilateral PQRS, if the bisectors of ∠ SPQ and ∠ PQR meet at O, prove that O is equidistant from PS and QR.
In Fig. ABCD is a quadrilateral in which AB = BC. E is the point of intersection of the right bisectors of AD and CD. Prove that BE bisects ∠ABC.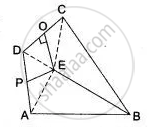
ΔPBC and ΔQBC are two isosceles triangles on the same base. Show that the line PQ is bisector of BC and is perpendicular to BC.
Find the locus of points which are equidistant from three non-collinear points.
Show that the locus of the centres of all circles passing through two given points A and B, is the perpendicular bisector of the line segment AB.
