Advertisements
Advertisements
प्रश्न
Explain why, for a charge configuration, the equipotential surface through a point is normal to the electric field at that point
उत्तर
For any charge configuration, the equipotential surface through a point is normal to the electric field at that point. If the field was not normal to the equipotential surface, it would have a non-zero component along the surface. To move a unit test charge against the direction of the component of the field, work would have to be done. However, this is in contradiction to the definition of an equipotential surface: there is no potential difference between any two points on the surface and no work is required to move a test charge on the surface. Therefore, the electric field must be normal to the equipotential surface at every point.
APPEARS IN
संबंधित प्रश्न
A 36 cm long sonometer wire vibrates with frequency of 280 Hz in fundamental mode, when it is under tension of 24.5 N. Calculate linear density of the material of wire.
"For any charge configuration, equipotential surface through a point is normal to the electric field." Justify.
Obtain the formula for the electric field due to a long thin wire of uniform linear charge density λ without using Gauss’s law. [Hint: Use Coulomb’s law directly and evaluate the necessary integral.]
Draw a graph to show the variation of E with perpendicular distance r from the line of charge.
State Gauss’Law.
If the ratio of radii of two wires of same material is 3 : 1 and ratio of their lengths is 5 : 1, then the ratio of the normal forces that will produce the same extension in the length of two wires is:
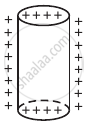
Sketch the electric field lines for a uniformly charged hollow cylinder shown in figure.
- Obtain the expression for the electric field intensity due to a uniformly charged spherical shell of radius R at a point distant r from the centre of the shell outside it.
- Draw a graph showing the variation of electric field intensity E with r, for r > R and r < R.
Draw a graph of kinetic energy as a function of linear charge density λ.
